Class 8 Maths Chapter 14 Factorisation All Exercise NCERT Solutions

Class 8 Maths Chapter 14 Factorisation All Exercise NCERT Solutions

• Class 8 Maths Factorisation Exercise 14.1
• Class 8 Maths Factorisation Exercise 14.2
• Class 8 Maths Factorisation Exercise 14.3

NCERT Solutions for Class 8 Maths Chapter 14 Factorisation Exercise 14.1

Ex 14.1 Class 8 Maths Question 1.
Find the common factors of the given terms.
(i) 12x, 36
(ii) 2y, 22xy
(iii) 14pq, 28p2q2
(iv) 2x, 3x2, 4
(v) 6abc, 24ab2, 12a2b
(vi) 16x3, -4x2, 32x
(vii) 10pq, 20qr, 30rp
(viii) 3x2y3, 10x3y2, 6x2y2z
Solution:
(i) 12x, 36
(2 × 2 × 3 × x) and (2 × 2 × 3 × 3)
Common factors are 2 × 2 × 3 = 12
Hence, the common factor = 12

(ii) 2y, 22xy
= (2 × y) and (2 × 11 × x × y)
Common factors are 2 × y = 2y
Hence, the common factor = 2y

(iii) 14pq, 28p2q2
= (2 × 7 × p × q) and (2 × 2 × 7 × p × p × q × q)
Common factors are 2 × 7 × p × q = 14pq
Hence, the common factor = 14pq

(iv) 2x, 3x2, 4
= (2 × x), (3 × x × x) and (2 × 2)
Common factor is 1
Hence, the common factor = 1 [∵ 1 is a factor of every number]

(v) 6abc, 24ab2, 12a2b
= (2 × 3 × a × b × c), (2 × 2 × 2 × 3 × a × b × b) and (2 × 2 × 3 × a × a × b)
Common factors are 2 × 3 × a × b = 6ab
Hence, the common factor = 6ab

(vi) 16x3, -4x2, 32x
= (2 × 2 × 2 × 2 × x × x × x), -(2 × 2 × x × x), (2 × 2 × 2 × 2 × 2 × x)
Common factors are 2 × 2 × x = 4x
Hence, the common factor = 4x

(vii) 10pq, 20qr, 30rp
= (2 × 5 × p × q), (2 × 2 × 5 × q × r), (2 × 3 × 5 × r × p)
Common factors are 2 × 5 = 10
Hence, the common factor = 10

(viii) 3x2y2, 10x3y2, 6x2y2z
= (3 × x × x × y × y), (2 × 5 × x × x × x × y × y), (2 × 3 × x × x × y × y × z)
Common factors are x × x × y × y = x2y2
Hence, the common factor = x2y2.

Ex 14.1 Class 8 Maths Question 2.
Factorise the following expressions.
(i) 7x – 42
(ii) 6p – 12q
(iii) 7a2 + 14a
(iv) -16z + 20z3
(v) 20l2m + 30alm
(vi) 5x2y – 15xy2
(vii) 10a2 – 15b2 + 20c2
(viii) -4a2 + 4ab – 4ca
(ix) x2yz + xy2z + xyz2
(x) ax2y + bxy2 + cxyz
Solution:
(i) 7x – 42 = 7(x – 6)
(ii) 6p – 12q = 6(p – 2q)
(iii) 7a2 + 14a = 7a(a + 2)
(iv) -16z + 20z3 = 4z(-4 + 5z2)
(v) 20l2m + 30alm = 10lm(2l + 3a)
(vi) 5x2y – 15xy2 = 5xy(x – 3y)
(vii) 10a2 – 15b2 + 20c2 = 5(2a2 – 3b2 + 4c2)
(viii) -4a2 + 4ab – 4ca = 4a(-a + b – c)
(ix) x2yz + xy2z + xyz2 = xyz(x + y + z)
(x) ax2y + bxy2 + cxyz = xy(ax + by + cz)

Ex 14.1 Class 8 Maths Question 3.
Factorise:
(i) x2 + xy + 8x + 8y
(ii) 15xy – 6x + 5y – 2
(iii) ax + bx – ay – by
(iv) 15pq + 15 + 9q + 25p
(v) z – 7 + 7xy – xyz
Solution:
(i) x2 + xy + 8x + 8y
Grouping the terms, we have
x2 + xy + 8x + 8y
= x(x + y) + 8(x + y)
= (x + y)(x + 8)
Hence, the required factors = (x + y)(x + 8)

(ii) 15xy – 6x + 5y – 2
Grouping the terms, we have
(15xy – 6x) + (5y – 2)
= 3x(5y – 2) + (5y – 2)
= (5y – 2)(3x + 1)

(iii) ax + bx – ay – by
Grouping the terms, we have
= (ax – ay) + (bx – by)
= a(x – y) + b(x – y)
= (x – y)(a + b)
Hence, the required factors = (x – y)(a + b)

(iv) 15pq + 15 + 9q + 25p
Grouping the terms, we have
= (15pq + 25p) + (9q + 15)
= 5p(3q + 5) + 3(3q + 5)
= (3q + 5) (5p + 3)
Hence, the required factors = (3q + 5) (5p + 3)

(v) z – 7 + 7xy – xyz
Grouping the terms, we have
= (-xyz + 7xy) + (z – 7)
= -xy(z – 7) + 1 (z – 7)
= (-xy + 1) (z – 1)
Hence the required factor = -(1 – xy) (z – 7)

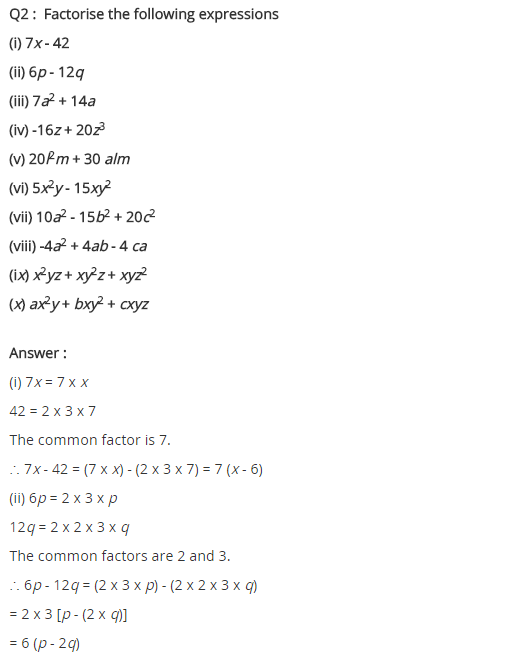
NCERT Solutions for Class 8 Maths Chapter 14 Factorisation Exercise 14.2

Ex 14.2 Class 8 Maths Question 1.
Factorise the following expressions.
(i) a2 + 8a +16
(ii) p2 – 10p + 25
(iii) 25m2 + 30m + 9
(iv) 49y2 + 84yz + 36z2
(v) 4x2 – 8x + 4
(vi) 121b2 – 88bc + 16c2
(vii) (l + m)2 – 4lm. (Hint: Expand (l + m)2 first)
(viii) a4 + 2a2b2 + b4
Solution:
(i) a2 + 8a + 16
Here, 4 + 4 = 8 and 4 × 4 = 16
a2 + 8a +16
= a2 + 4a + 4a + 4 × 4
= (a2 + 4a) + (4a + 16)
= a(a + 4) + 4(a + 4)
= (a + 4) (a + 4)
= (a + 4)2

(ii) p2 – 10p + 25
Here, 5 + 5 = 10 and 5 × 5 = 25
p2 – 10p + 25
= p2 – 5p – 5p + 5 × 5
= (p2 – 5p) + (-5p + 25)
= p(p – 5) – 5(p – 5)
= (p – 5) (p – 5)
= (p – 5)2

(iii) 25m2 + 30m + 9
Here, 15 + 15 = 30 and 15 × 15 = 25 × 9 = 225
25m2 + 30m + 9
= 25m2 + 15m + 15m + 9
= (25m2 + 15m) + (15m + 9)
= 5m(5m + 3) + 3(5m + 3)
= (5m + 3) (5m + 3)
= (5m + 3)2

(iv) 49y2 + 84yz + 36z2
Here, 42 + 42 = 84 and 42 × 42 = 49 × 36 = 1764
49y2 + 84yz + 36z2
= 49y2 + 42yz + 42yz + 36z2
= 7y(7y + 6z) +6z(7y + 6z)
= (7y + 6z) (7y + 6z)
= (7y + 6z)2

(v) 4x2 – 8x + 4
= 4(x2 – 2x + 1) [Taking 4 common]
= 4(x2 – x – x + 1)
= 4[x(x – 1) -1(x – 1)]
= 4(x – 1)(x – 1)
= 4(x – 1)2

(vi) 121b2 – 88bc + 16c2
Here, 44 + 44 = 88 and 44 × 44 = 121 × 16 = 1936
121b2 – 88bc + 16c2
= 121b2 – 44bc – 44bc + 16c2
= 11b(11b – 4c) – 4c(11b – 4c)
= (11b – 4c) (11b – 4c)
= (11b – 4c)2

(vii) (l + m)2 – 4lm
Expanding (l + m)2, we get
l2 + 2lm + m2 – 4lm
= l2 – 2lm + m2
= l2 – Im – lm + m2
= l(l – m) – m(l – m)
= (l – m) (l – m)
= (l – m)2

(viii) a4 + 2a2b2 + b4
= a4 + a2b2 + a2b2 + b4
= a2(a2 + b2) + b2(a2 + b2)
= (a2 + b2)(a2 + b2)
= (a2 + b2)2

Ex 14.2 Class 8 Maths Question 2.
Factorise.
(i) 4p2 – 9q2
(ii) 63a2 – 112b2
(iii) 49x2 – 36
(iv) 16x5 – 144x3
(v) (l + m)2 – (l – m)2
(vi) 9x2y2 – 16
(vii) (x2 – 2xy + y2) – z2
(viii) 25a2 – 4b2 + 28bc – 49c2
Solution:
(i) 4p2 – 9q2
= (2p)2 – (3q)2
= (2p – 3q) (2p + 3q)
[∵ a2 – b2 = (a + b)(a – b)]

(ii) 63a2 – 112b2
= 7(9a2 – 16b2)
= 7 [(3a)2 – (4b)2]
= 7(3a – 4b)(3a + 4b)
[∵ a2 – b2 = (a + b)(a – b)]

(iii) 49x2 – 36 = (7x)2 – (6)2
= (7x – 6) (7x + 6)
[∵ a2 – b2 = (a + b)(a – b)]

(iv) 16x5 – 144x3 = 16x3 (x2 – 9)
= 16x3 [(x)2 – (3)2]
= 16x3(x – 3)(x + 3)
[∵ a2 – b2 = (a + b)(a – b)]

(v) (l + m)2 – (l – m)2
= (l + m) – (l – m)] [(l + m) + (l – m)]
[∵ a2 – b2 = (a + b)(a – b)]
= (l + m – l + m)(l + m + l – m)
= (2m) (2l)
= 4ml

(vi) 9x2y2 – 16 = (3xy)2 – (4)2
= (3xy – 4)(3xy + 4)
[∵ a2 – b2 = (a + b)(a – b)]

(vii) (x2 – 2xy + y2) – z2
= (x – y)2 – z2
= (x – y – z) (x – y + z)
[∵ a2 – b2 = (a + b)(a – b)]

(viii) 25a2 – 4b2 + 28bc – 49c2
= 25a2 – (4b2 – 28bc + 49c2)
= (5a)2 – (2b – 7c)2
= [5a – (2b – 7c)] [5a + (2b – 7c)]
= (5a – 2b + 7c)(5a + 2b – 7c)

Ex 14.2 Class 8 Maths Question 3.
Factorise the expressions.
(i) ax2 + bx
(ii) 7p2 + 21q2
(iii) 2x3 + 2xy2 + 2xz2
(iv) am2 + bm2 + bn2 + an2
(v) (lm + l) + m + 1
(vi) y(y + z) + 9(y + z)
(vii) 5y2 – 20y – 8z + 2yz
(viii) 10ab + 4a + 5b + 2
(ix) 6xy – 4y + 6 – 9x
Solution:
(i) ax2 + bx = x(ax + 5)

(ii) 7p2 + 21q2 = 7(p2 + 3q2)

(iii) 2x3 + 2xy2 + 2xz2 = 2x(x2 + y2 + z2)

(iv) am2 + bm2 + bn2 + an2
= m2 (a + b) + n2(a + b)
= (a + b)(m2 + n2)

(v) (lm + l) + m + 1
= l(m + 1) + (m + 1)
= (m + 1) (l + 1)

(vi) y(y + z) + 9(y + z) = (y + z)(y + 9)

(vii) 5y2 – 20y – 8z + 2yz
= 5y2 – 20y + 2yz – 8z
= 5y(y – 4) + 2z(y – 4)
= (y – 4) (5y + 2z)

(viii) 10ab + 4a + 5b + 2
= 2a(5b + 2) + 1(5b + 2)
= (5b + 2)(2a + 1)

(ix) 6xy – 4y + 6 – 9x
= 6xy – 4y – 9x + 6
= 2y(3x – 2) – 3(3x – 2)
= (3x – 2) (2y – 3)

Ex 14.2 Class 8 Maths Question 4.
Factorise.
(i) a4 – b4
(ii) p4 – 81
(iii) x4 – (y + z)4
(iv) x4 – (x – z)4
(v) a4 – 2a2b2 + b4
Solution:
(i) a4 – b4 – (a2)2 – (b2)2
[∵ a2 – b2 = (a – b)(a + b)]
= (a2 – b2) (a2 + b2)
= (a – b) (a + b) (a2 + b2)

(ii) p4 – 81 = (p2)2 – (9)2
= (p2 – 9) (p2 + 9)
[∵ a2 – b2 = (a – b)(a + b)]
= (p – 3)(p + 3) (p2 + 9)

(iii) x4 – (y + z)4 = (x2)2 – [(y + z)2]2
[∵ a2 – b2 = (a – b)(a + b)]
= [x2 – (y + z)2] [x2 + (y + z)2]
= [x – (y + z)] [x + (y + z)] [x2 + (y + z)2]
= (x – y – z) (x + y + z) [x2 + (y + z)2]

(iv) x4 – (x – z)4 = (x2)2 – [(y – z)2]2
= [x2 – (y – z)2] [x2 + (y – z)2]
= (x – y + z) (x + y – z) (x2 + (y – z)2]

(v) a4 – 2a2b2 + b4
= a4 – a2b2 – a2b2 + b4
= a2(a2 – b2) – b2(a2 – b2)
= (a2 – b2)(a2 – b2)
= (a2 – b2)2
= [(a – b) (a + b)]2
= (a – b)2 (a + b)2

Ex 14.2 Class 8 Maths Question 5.
Factorise the following expressions.
(i) p2 + 6p + 8
(ii) q2 – 10q + 21
(iii) p2 + 6p – 16
Solution:
(i) p2 + 6p + 8
Here, 2 + 4 = 6 and 2 × 4 = 8
p2 + 6p + 8
= p2 + 2p + 4p + 8
= p (p + 2) + 4(p + 2)
= (p + 2) (p + 4)

(ii) q2 – 10q + 21
Here, 3 + 7 = 10 and 3 × 7 = 21
q2 – 10q + 21
= q2 – 3q – 7q + 21
= q(q – 3) – 7(q – 3)
= (q – 3) (q – 7)

(iii) p2 + 6p – 16
Here, 8 – 2 = 6 and 8 × 2 = 16
p2 + 6p – 16
= p2 + 8p – 2p – 16
= p(p + 8) – 2(p + 8)
= (p + 8) (p – 2)

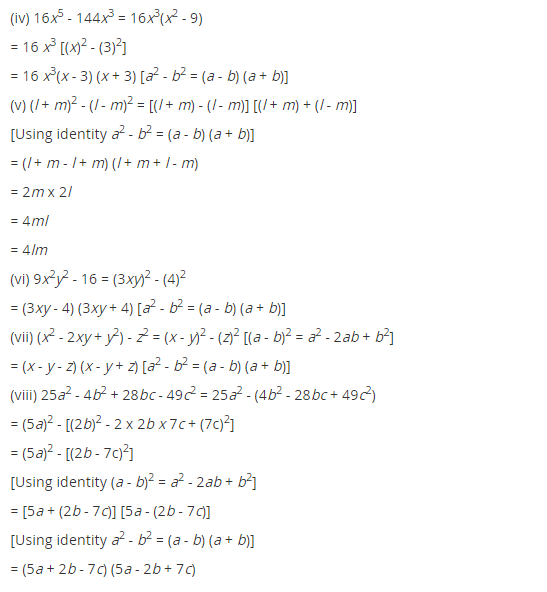
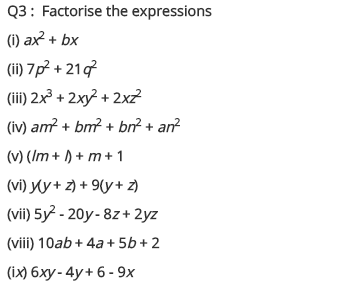
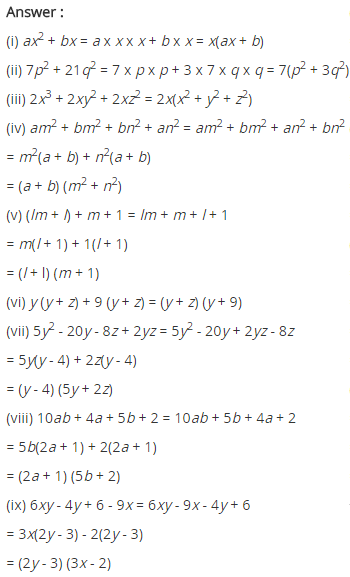
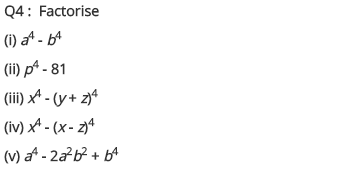
NCERT Solutions for Class 8 Maths Chapter 14 Factorisation Exercise 14.3

Ex 14.3 Class 8 Maths Question 1.
Carry out the following divisions.
(i) 28x4 ÷ 56x
(ii) -36y3 ÷ 9y2
(iii) 66pq2r3 ÷ 11qr2
(iv) 34x3y3z3 ÷ 51xy2z3
(v) 12a8b8 ÷ (-6a6b4)
Solution: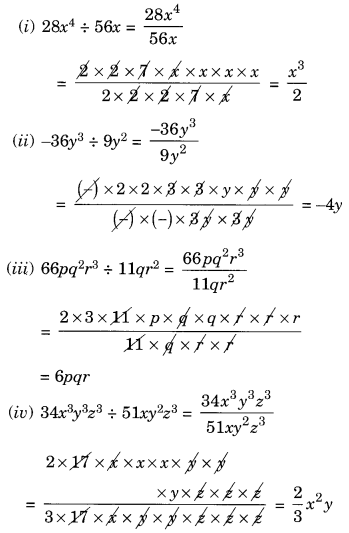

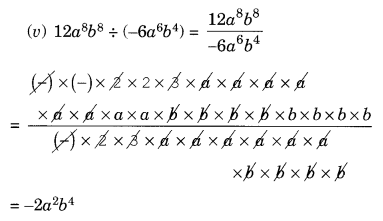
Ex 14.3 Class 8 Maths Question 2.
Divide the following polynomial by the given monomial.
(i) (5x2 – 6x) ÷ 3x
(ii) (3y8 – 4y6 + 5y4) ÷ y4
(iii) 8(x3y2z2 + x2y3z2 + x2y2z3) ÷ 4x2y2z2
(iv) (x3 + 2x2 + 3x) ÷ 2x
(v) (p3q6 – p6q3) ÷ p3q3
Solution: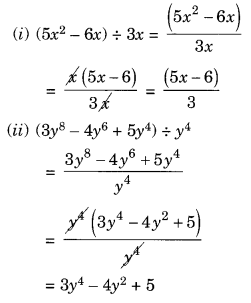

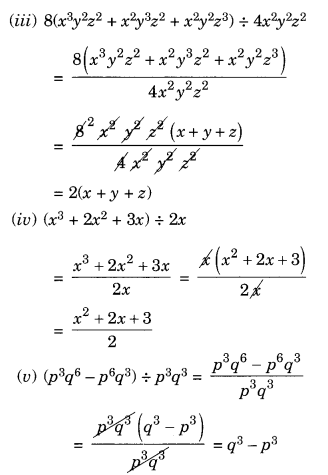
Ex 14.3 Class 8 Maths Question 3.
Work out the following divisions.
(i) (10x – 25) ÷ 5
(ii) (10x – 25) ÷ (2x – 5)
(iii) 10y(6y + 21) ÷ 5(2y + 7)
(iv) 9x2y2(3z – 24) ÷ 27xy(z – 8)
(v) 96abc(3a – 12) (5b – 30) ÷ 144(a – 4)(b – 6)
Solution: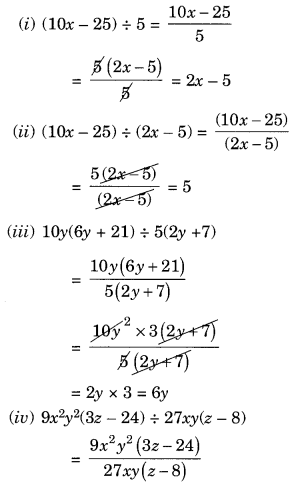

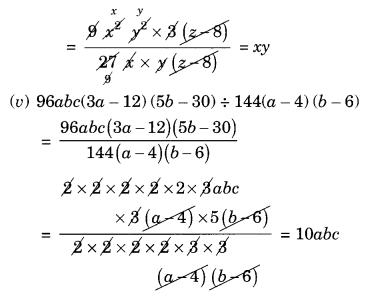
Ex 14.3 Class 8 Maths Question 4.
Divide as directed.
(i) 5(2x + 1) (3x + 5) ÷ (2x + 1)
(ii) 26xy (x + 5)(y – 4) ÷ 13x(y – 4)
(iii) 52pqr(p + q) (q + r) (r + p) ÷ 104pq(q + r)(r + p)
(iv) 20(y + 4)(y2 + 5y + 3) ÷ 5(y + 4)
(v) x(x + 1) (x + 2) (x + 3) ÷ x(x + 1)
Solution: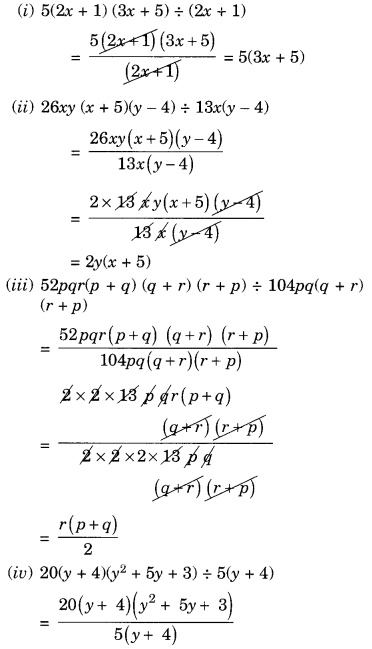

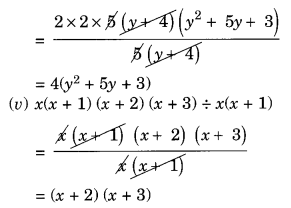
Ex 14.3 Class 8 Maths Question 5.
Factorise the expressions and divide them as directed.
(i) (y2 + 7y + 10) ÷ (y + 5)
(ii) (m2 – 14m – 32) ÷ (m + 2)
(iii) (5p2 – 25p + 20) ÷ (p – 1)
(iv) 4yz(z2 + 6z – 16) ÷ 2y(z + 8)
(v) 5pq(p2 – q2) ÷ 2p(p + q)
(vi) 12xy(9x2 – 16y2) ÷ 4xy(3x + 4y)
(vii) 39y3(50y2 – 98) ÷ 26y2(5y + 7)
Solution:

NCERT Solutions for Class 8 Maths

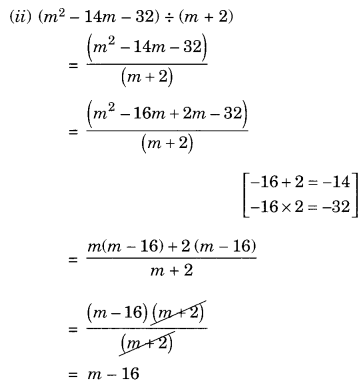
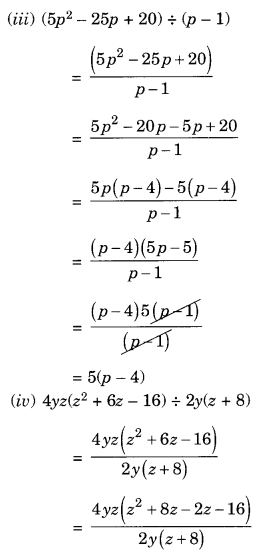
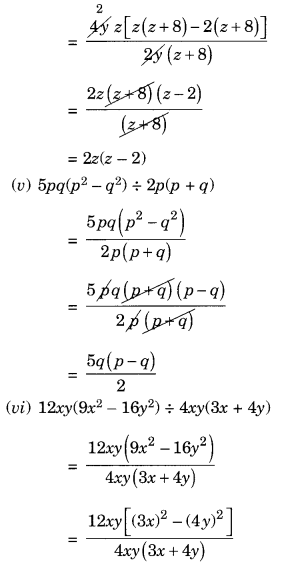
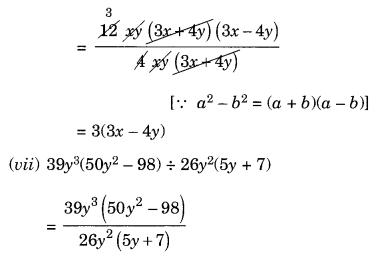
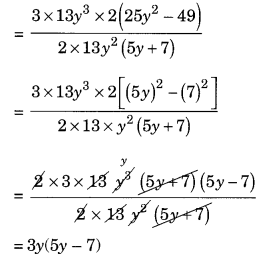
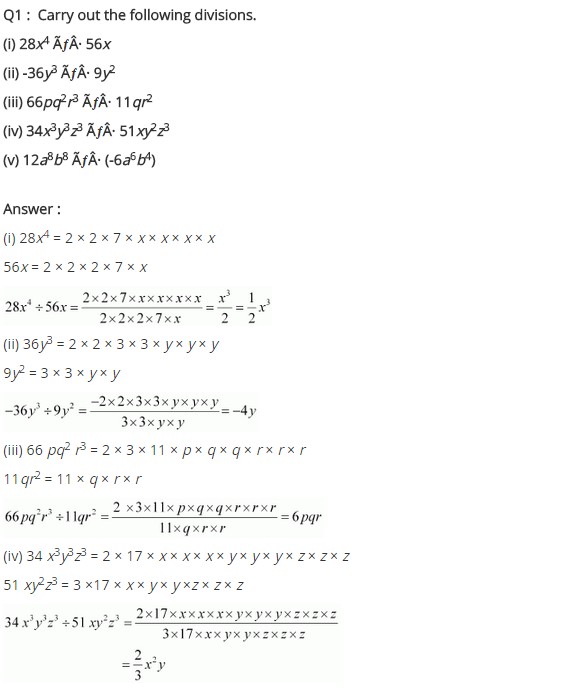
• Chapter 1 Rational Numbers
• Chapter 2 Linear Equations in One Variable
• Chapter 3 Understanding Quadrilaterals
• Chapter 4 Practical Geometry
• Chapter 5 Data Handling
• Chapter 6 Squares and Square Roots
• Chapter 7 Cubes and Cube Roots
• Chapter 8 Comparing Quantities
• Chapter 9 Algebraic Expressions and Identities
• Chapter 10 Visualising Solid Shapes
• Chapter 11 Mensuration
• Chapter 12 Exponents and Powers
• Chapter 13 Direct and Indirect proportions
• Chapter 14 Factorisation
• Chapter 15 Introduction to Graphs
• Chapter 16 Playing with Numbers