Class 10 Maths Chapter 12 Areas Related to Circles (English + Hindi) NCERT Solutions

Class 10 Maths Chapter 12 Areas Related to Circles (English + Hindi) NCERT Solutions

Table Of Conent

• Ex.12.1
• Ex.12.2
• Ex.12.3
• Applications of Trigonometry Class 10 Extra Questions
• Some Applications of Trigonometry Class 10 Important Questions
• Some Applications of Trigonometry Class 10 Notes

Ex 12.1

Ex 12.1 Class 10 Maths Question 1.
The radii of the two circles are 19 cm and 9 cm respectively. Find the radius of the circle which has a circumference equal to the sum of the circumferences of the two circles.
Solution:

Ex 12.1 Class 10 Maths Question 2.
The radii of two circles are 8 cm and 6 cm respectively. Find the radius of the circle having area equal to the sum of the areas of the two circles.
Solution: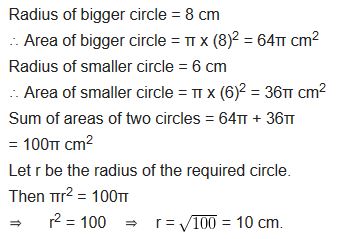

You can also download the free PDF of  Ex 12.1 Class 10 Areas Related to Circles NCERT Solutions or save the solution images and take the print out to keep it handy for your exam preparation.

Download NCERT Solutions For Class 10 Maths Chapter 12 Areas Related to Circles PDF

Ex 12.1 Class 10 Maths Question 3.
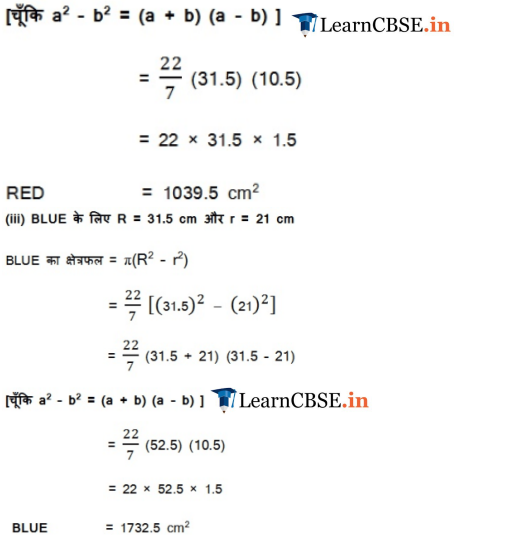
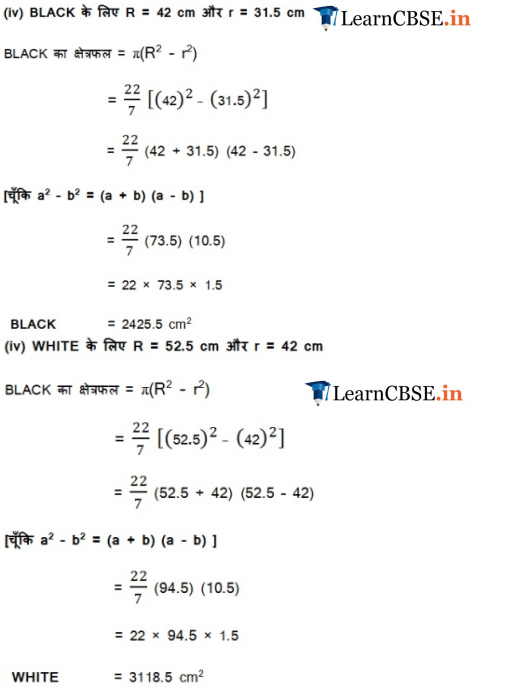
The given figure depicts an archery target marked with its five scoring regions from the center outwards as Gold, Red, Blue, Black, and White.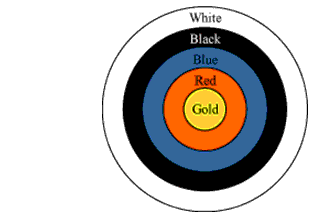

The diameter of the region representing Gold score is 21 cm and each of the other bands is 10.5 cm wide. Find the area of each of the five scoring regions.
Solution:

Formulae Handbook for Class 10 Maths and Science

Ex 12.1 Class 10 Maths Question 4.
The wheels of a car are of diameter 80 cm each. How many complete revolutions does each wheel make in 10 minutes when the car is travelling at a speed of 66 km per hour?
Solution:

Ex 12.1 Class 10 Maths Question 5.
Tick the correct answer in the following and justify your choice: If the perimeter and the area of a circle are numerically equal, then the radius of the circle is
(a) 2 units
(b) n units
(c) 4 units
(d) 7 units
Solution:

NCERT Solutions for Class 10 Maths Chapter 12 Areas Related to Circles (Hindi Medium) Ex 12.1

Class 10 Maths Areas Related To Circles Mind Maps

Terms Related To Circle

(i) Chord: A line segment joining any two points on a circle.
(ii) Arc: A piece of a circle between two points on the circle is called an arc.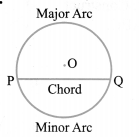

The arc less than the semicircular arc is called minor arc and the one greater than the semi-circular arc is called major arc.
(iii) Sector: The portion of a circular region enclosed by two radii and the corresponding arc is called a sector of the circle.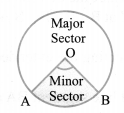

Sector smaller than the semi-circle is called minor sector and the sector larger than the semi-circle is called major sector.
(iv) Segment: The portion of a circular region enclosed between a chord and the corresponding arc is called a segment of the circle.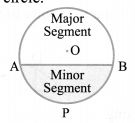

The segment bounded by the chord and the minor arc intercepted by the chord is called minor segment and the segment bounded by the chord and the major arc intercepted by the chord is called major segment.

Circle

The set of all points in a plane which are at a fixed distance from a fixed point in the plane is called circle. The fixed point is called centre and the fixed distance is called radius of the circle

Circumference and Area of a Circle
(i) The circumference of a circle is defined as distance covered by travelling once around a circle and is given by C = 2πr = πd
where r = radius of the circle and d = diameter of the circle.
(ii) The Area of a circle of radius r is given by,
A = πr2=π4d2 where, d = diameter of the circle.
(iii) Area of a circular ring:
The area of the circular path or ring is given by the difference of the area of outer circle and the area of inner circle.
Area of circular ring = n(R2 – r2)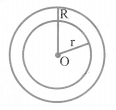

Length of an Arc and Area of Sector

(i) The length of an arc of a sector of an angle 9 is given by,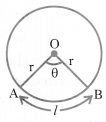

(ii) The area of the sector AOB of angle 0 is given by,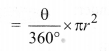

This is the area of minor sector.
∴ area of major sector AOB
= πr2 – Area of a minor sector AOB

Area of Segment

(i) Area of segment APB = Area (sector OAPB) – Area(∆OAB)
This is the area of minor segment.
∴ area of major segment AQB = πr2 – Area of minor segment APB

(ii) If θ is the central angle, then the area of segment APB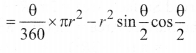

Class 10 Maths Chapter 12 Areas Related to Circles Ex 12.2

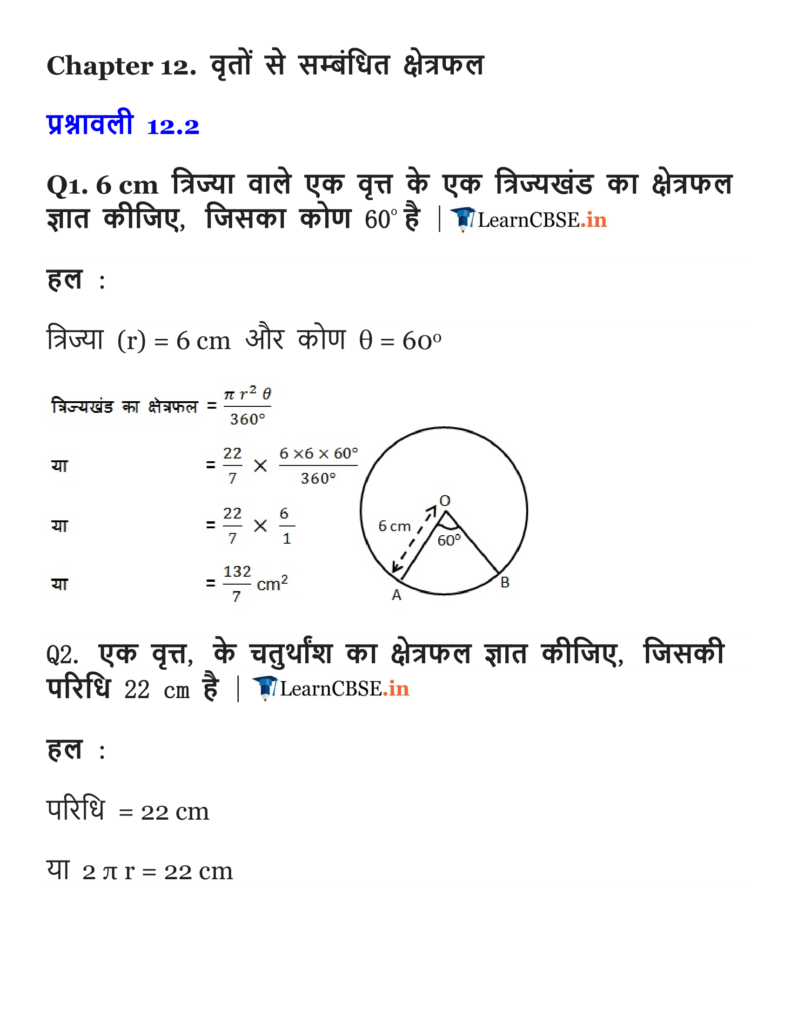
Ex 12.2 Class 10 Maths Question 1.

Find the area of a sector of a circle with radius 6 cm if angle of the sector is 60°.
Solution:

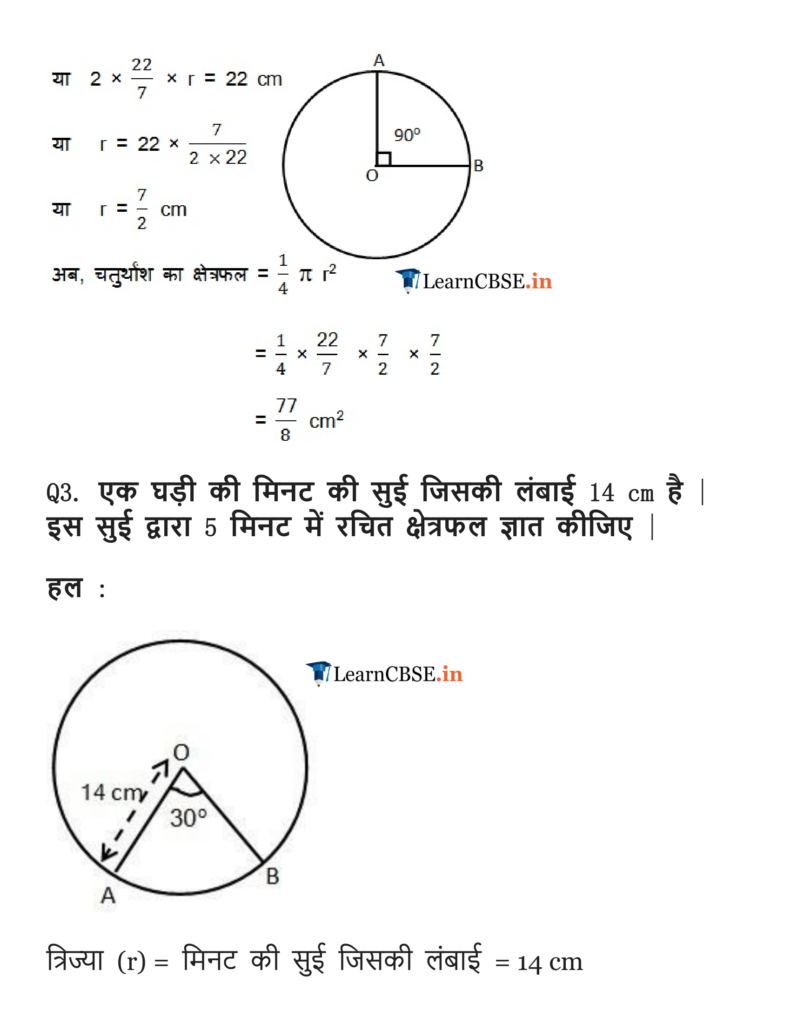
Ex 12.2 Class 10 Maths Question 2.
Find the area of a quadrant of a circle whose circumference is 22 cm.
Solution: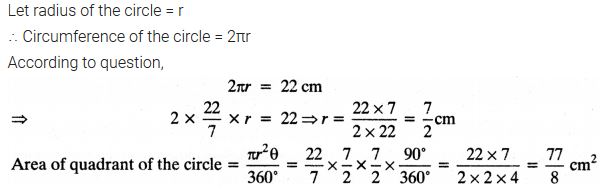

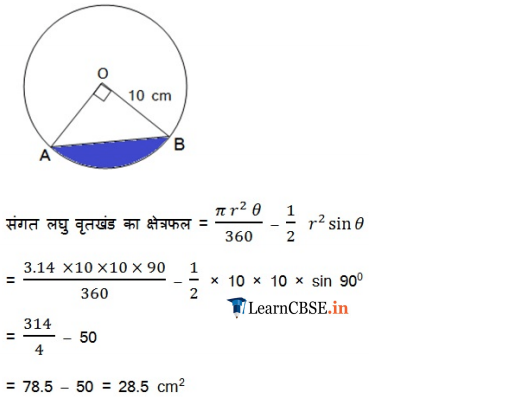
Ex 12.2 Class 10 Maths Question 3.

The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes.
Solution:

Ex 12.2 Class 10 Maths Question 4.
A chord of a circle of radius 10 cm subtends a right angle at the centre. Find the area of the corresponding:
(i) minor segment
(ii) major segment (Use π = 3.14)
Solution: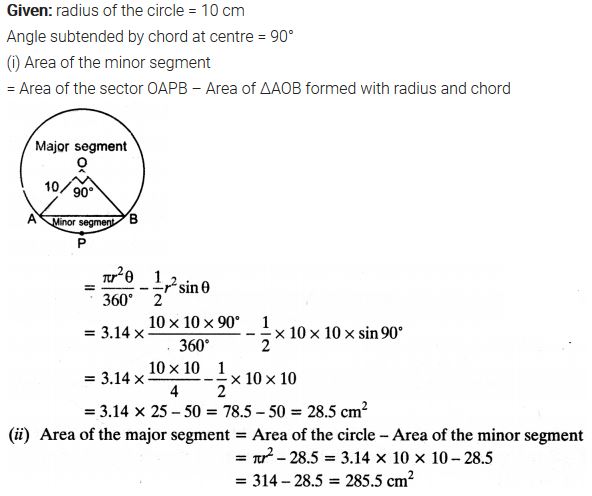

Ex 12.2 Class 10 Maths Question 5.
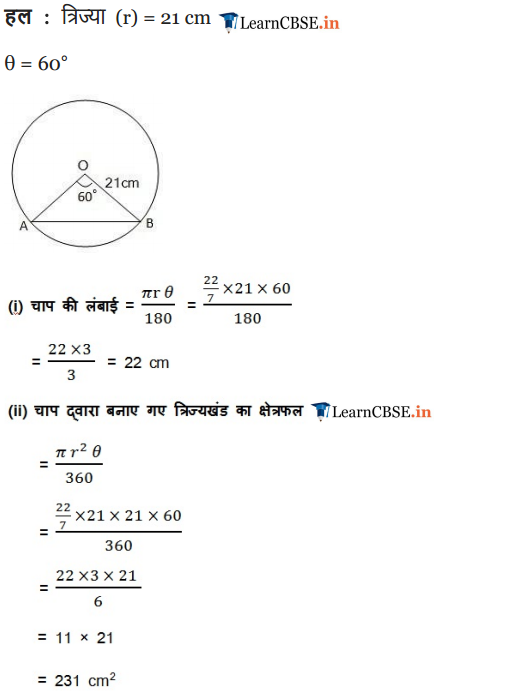
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find:
(i) length of the arc.
(ii) area of the sector formed by the arc.
(iii) area of the segment formed by the corresponding chord.
Solution: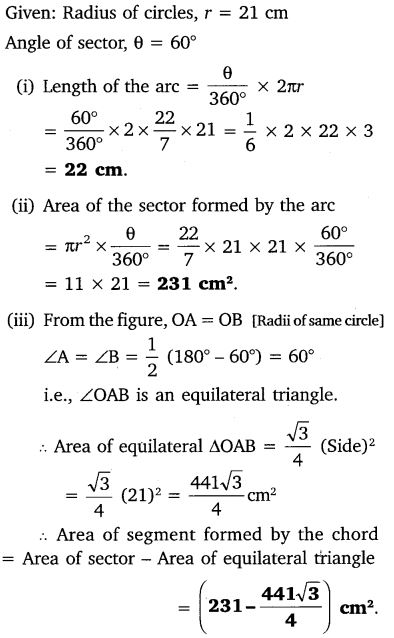

Ex 12.2 Class 10 Maths Question 6.
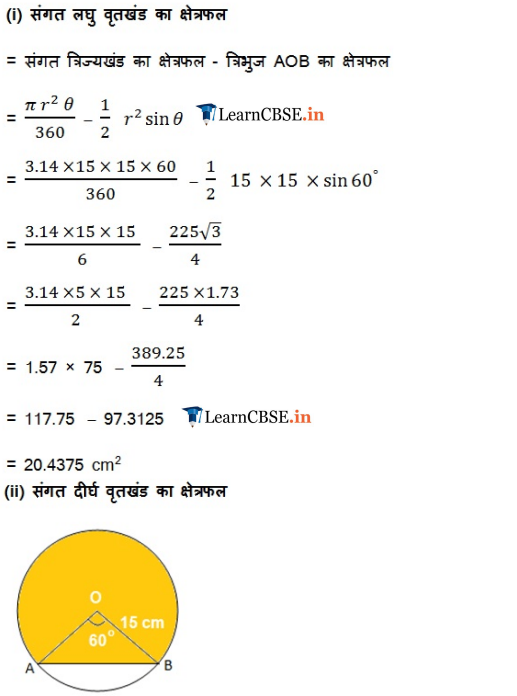
A chord of a circle of radius 15 cm subtends an angle of 60° at the centre. Find the areas of the corresponding minor and major segments of the circle. (Use π = 3.14 and √3 = 1.73)
Solution: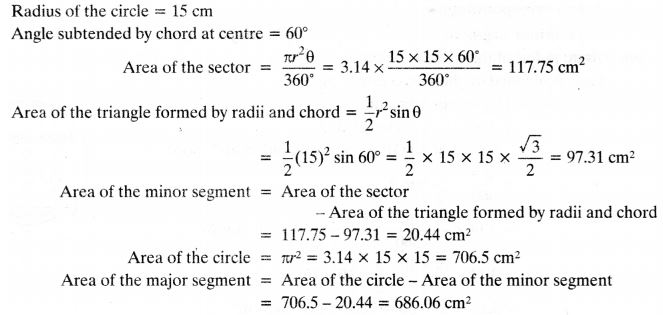

Ex 12.2 Class 10 Maths Question 7.
A chord of a circle of the radius 12 cm subtends an angle of 120° at the centre. Find the area of the corresponding segment of the circle. (Use π = 3.14 and √3 = 1.73).
Solution: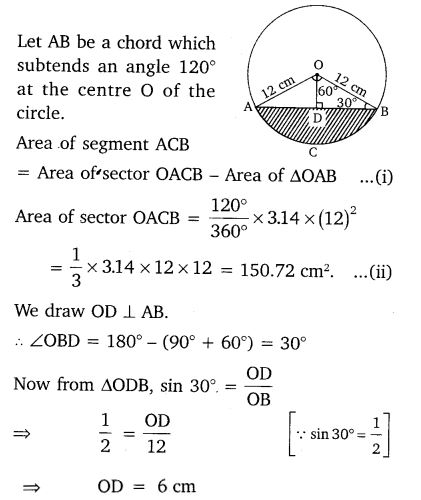

Ex 12.2 Class 10 Maths Question 8.
A horse is tied to a peg at one corner of a square shaped grass field of side 15 m by means of a 5 m long rope (see figure). Find: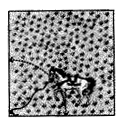

(i) the area of that part of the field in which the horse can graze.
(ii) the increase in the grazing area if the rope were 10 m long instead of 5 m. (Use π = 3.14)
Solution: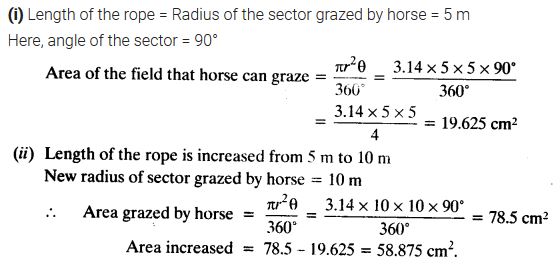

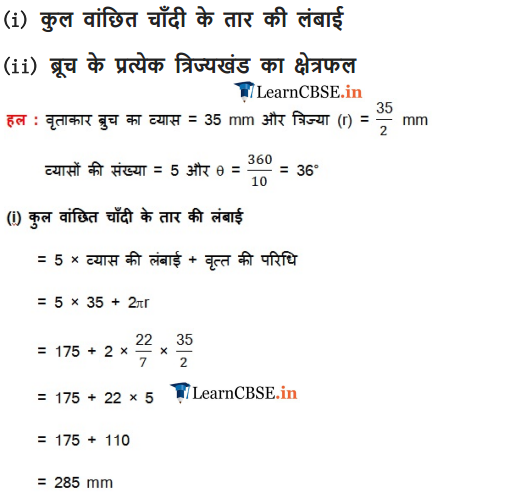
Ex 12.2 Class 10 Maths Question 9.
A brooch is made with silver wire in the form of a circle with diameter 35 mm. The wire is also used in making 5 diameters which divide the circle into 10 equal sectors as shown in figure.
Find: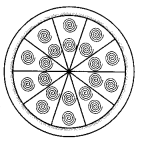

(i) the total length of the silver wire required.
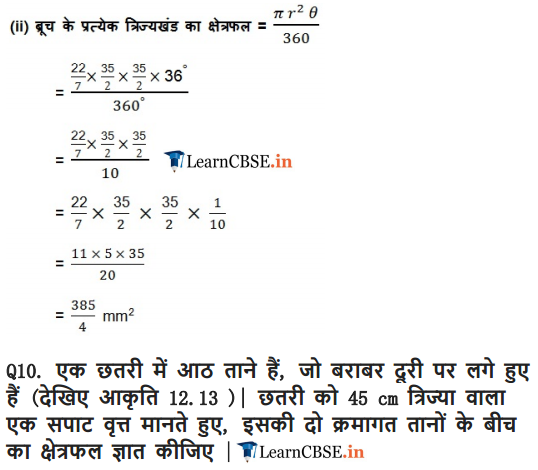
(ii) the area of each sector of the brooch.
Solution:

Ex 12.2 Class 10 Maths Question 10.
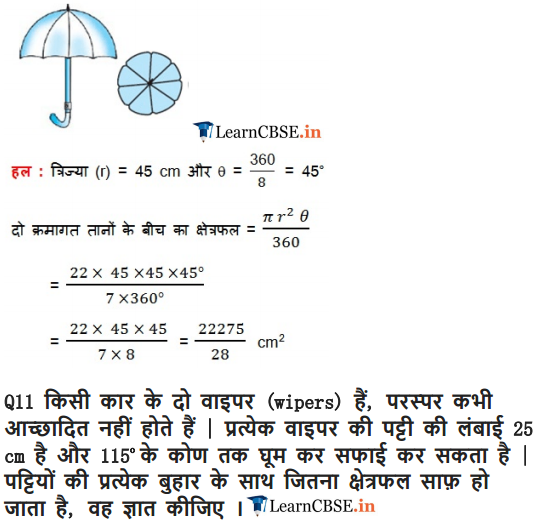
An umbrella has 8 ribs which are equally spaced (see figure). Assuming umbrella to be a flat circle of radius / 45 cm, find the area between the two consecutive ribs of the umbrella.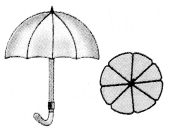

Solution: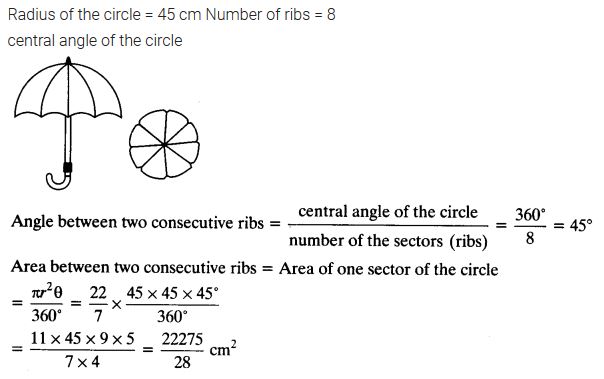

Ex 12.2 Class 10 Maths Question 11.
A car has two wipers which do not overlap.
Each wiper has a blade of length 25 cm sweeping through an angle of 115°. Find the total area cleaned at each sweep of the blades.
Solution: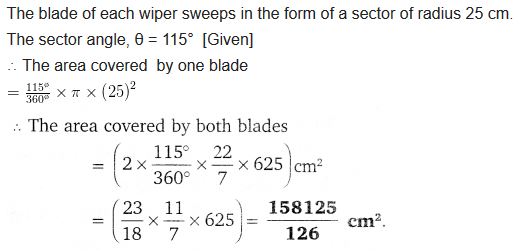

Ex 12.2 Class 10 Maths Question 12.
To warn ships for underwater rocks, a lighthouse spreads a red colored light over a sector of angle 80° to a distance of 16.5 km. Find the area of the sea over which the ships are warned. (Use π = 3.14)
Solution:

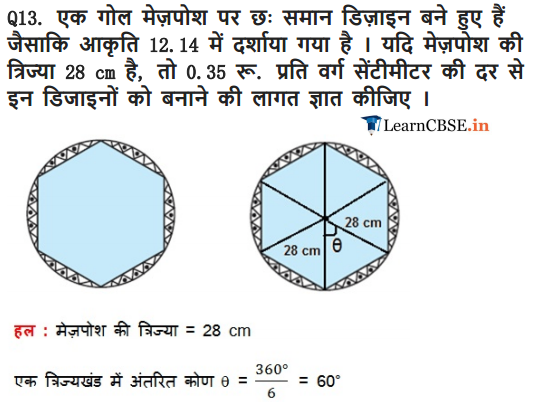
Ex 12.2 Class 10 Maths Question 13.
A round table cover has six equal designs as shown in the figure. If the radius of the cover is 28 cm, find the cost of making the designs at the rate of ₹0.35 per cm². (Use √3= 1.7)

Solution: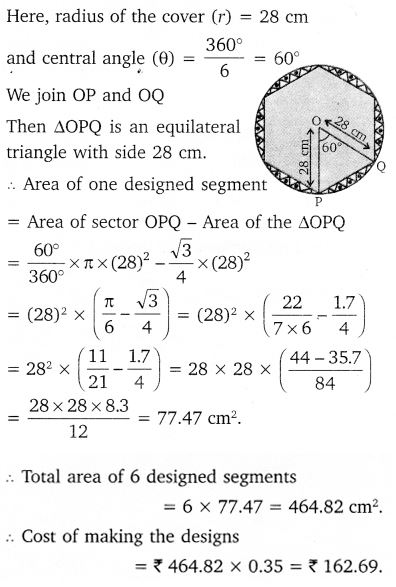

Ex 12.2 Class 10 Maths Question 14.
Tick the correct answer in the following: Area of a sector of angle p (in degrees) of a circle with radius R is

Solution:

NCERT Solutions for Class 10 Maths Chapter 12 Areas Related to Circles (Hindi Medium) Ex 12.2

Class 10 Maths Chapter 12 Areas Related to Circles Ex 12.3

[Unless stated otherwise, use 

π=227.]

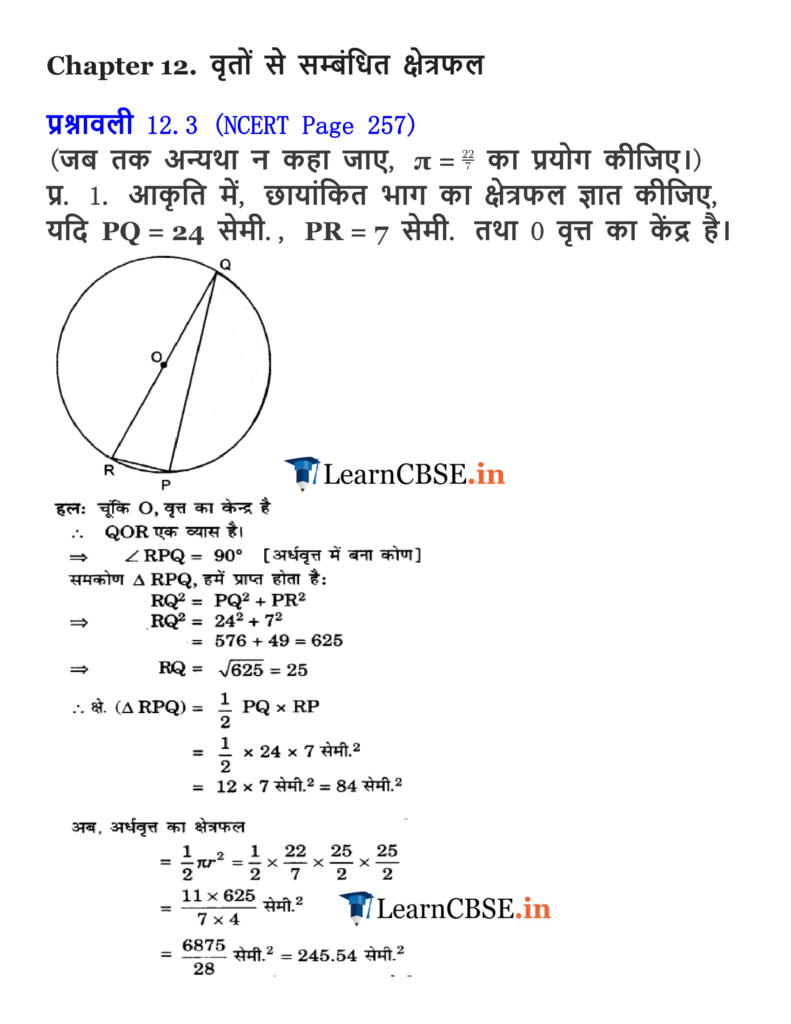
Ex 12.3 Class 10 Maths Question 1.
Find the area of the shaded region in the given figure, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle.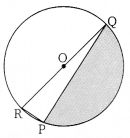

Solution:

Ex 12.3 Class 10 Maths Question 2.
Find the area of the shaded region in the given figure, ¡f radii of the two concentric circles with centre O are 7 cm and 14 cm respectively and ∠AOC = 40°.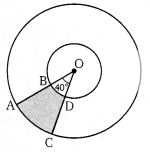

Solution: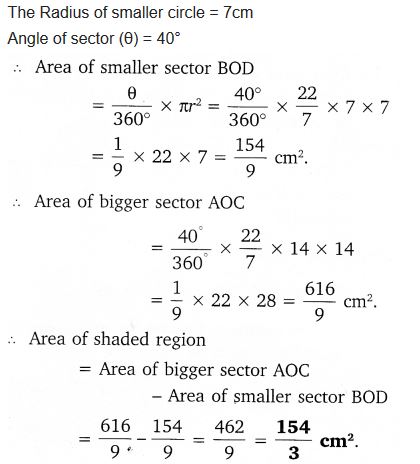

Ex 12.3 Class 10 Maths Question 3.
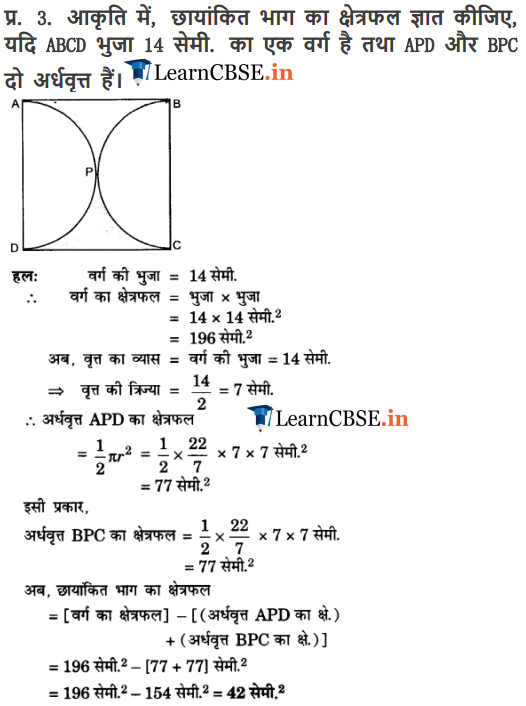
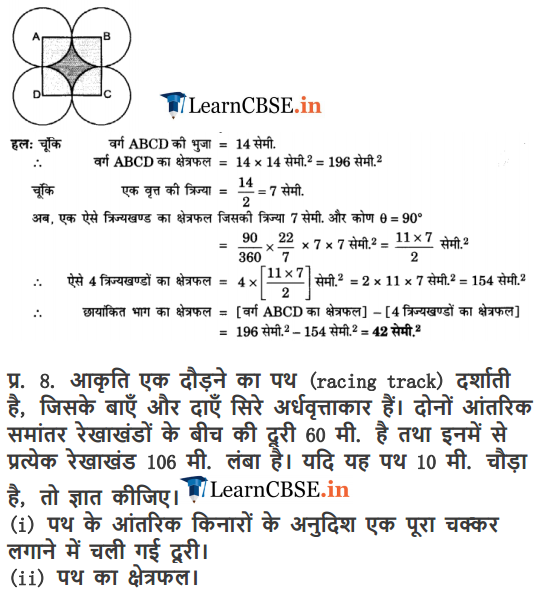
Find the area of the shaded region in the given figure, if ABCD is a square of side 14 cm and APD and BPC are semicircles.

Solution: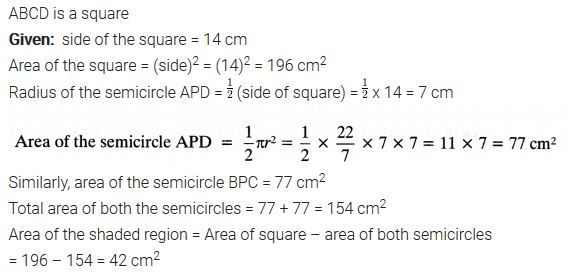

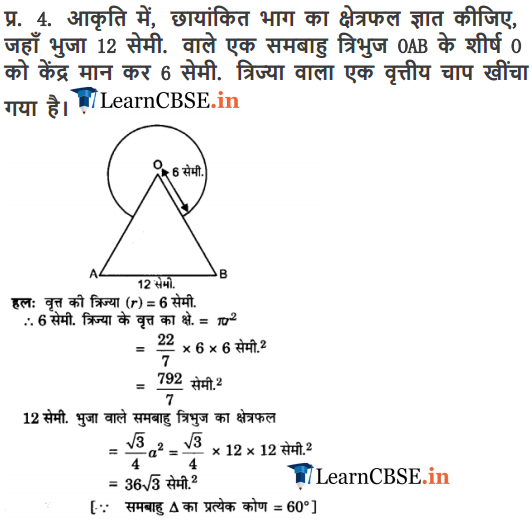
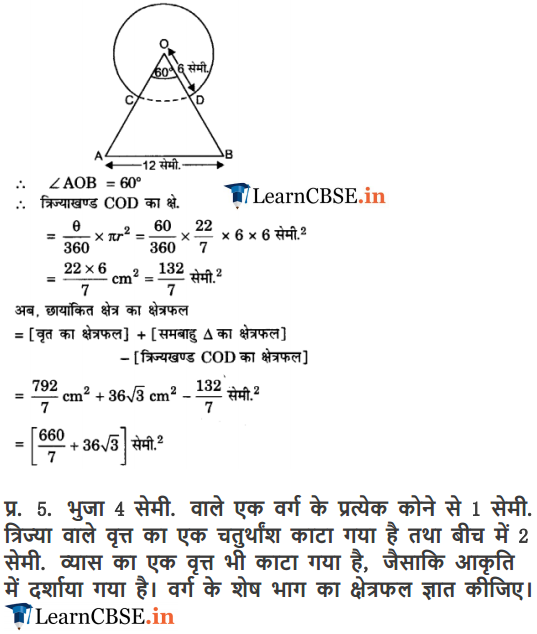
Ex 12.3 Class 10 Maths Question 4.
Find the area of the shaded region in the figure, where a circular arc of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm as centre.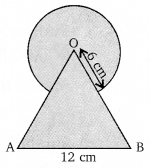

Solution: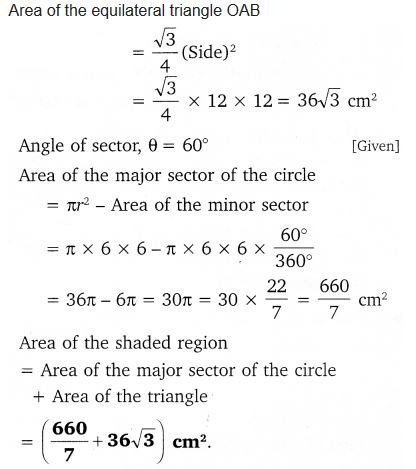

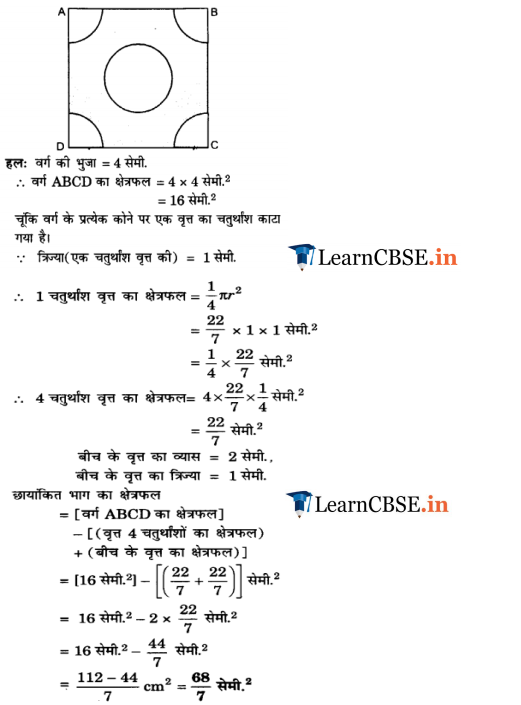
Ex 12.3 Class 10 Maths Question 5.
From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in the figure. Find the area of the remaining portion of the square.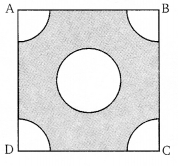

Solution: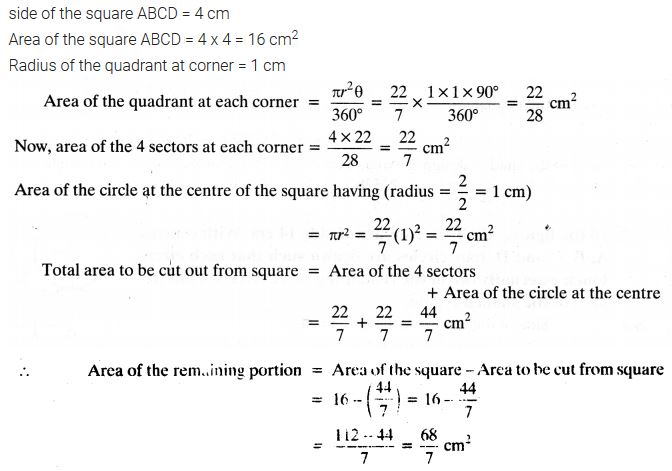

Ex 12.3 Class 10 Maths Question 6.
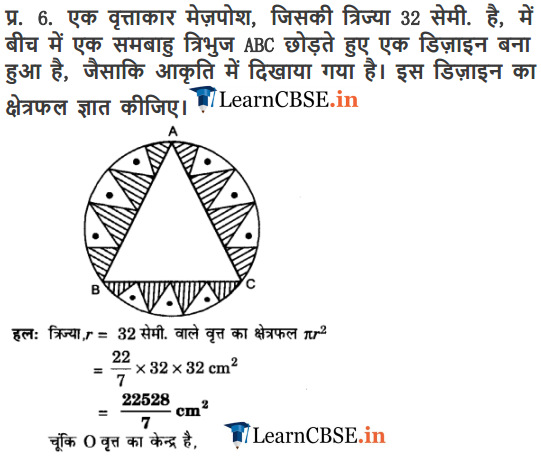
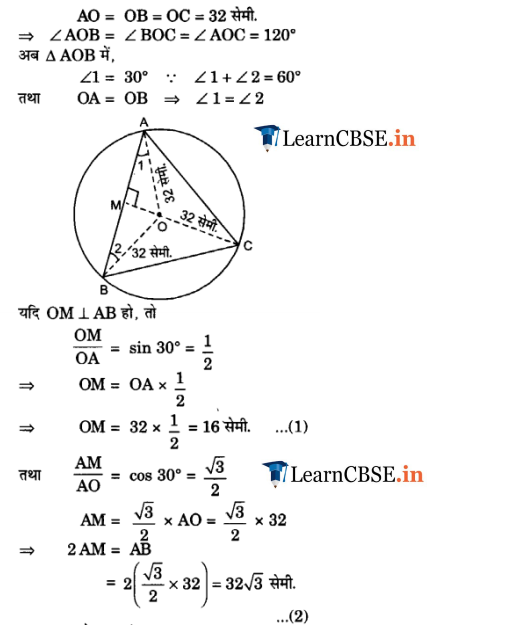
In a circular table cover of (he radius 32 cm, a design Is formed leaving an equilìtcral triangle ABC in the middle as shown in the figure. Find the area of the design (shaded region).

Solution: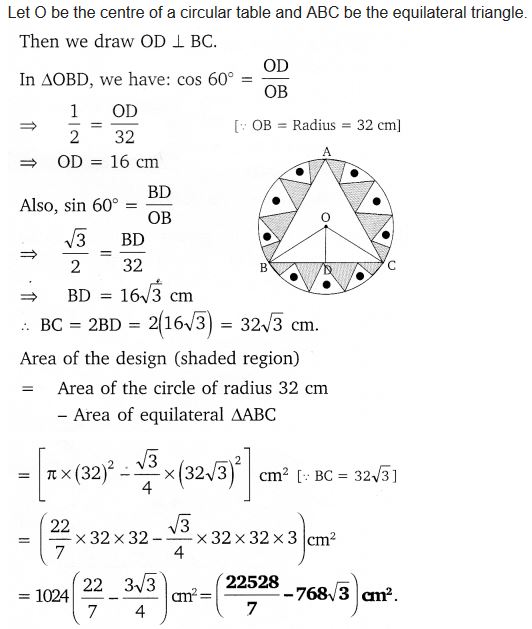

Ex 12.3 Class 10 Maths Question 7.
In the figure, ABCD is a square of side 14 cm. With centres A, B, C and D, four circles are drawn such that each circle touch externally two of the remaining three circles. Find the area of the shaded region.
Solution: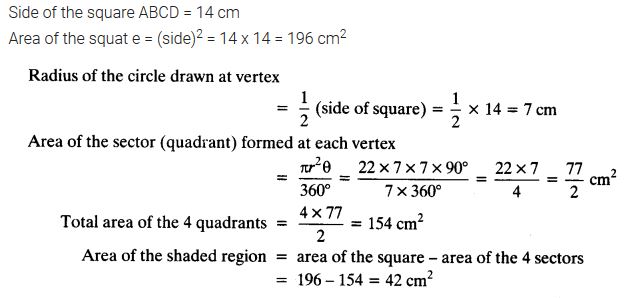

Ex 12.3 Class 10 Maths Question 8.
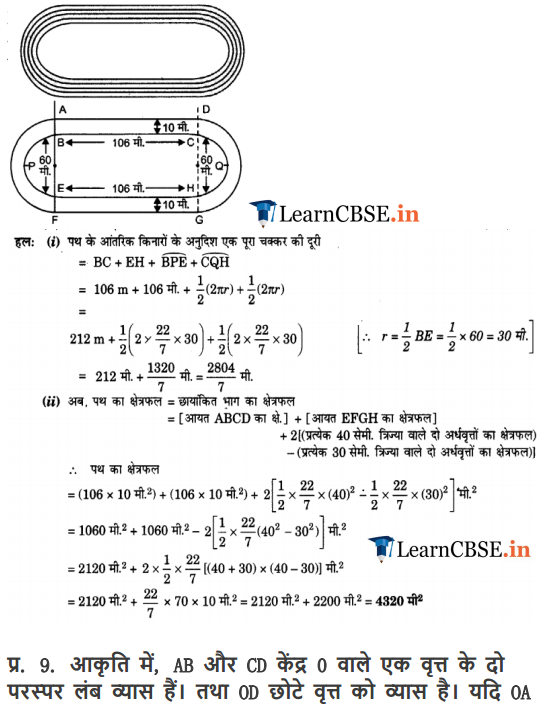
The given figure depicts a racing track whose left and right ends are semicircular. The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find:

(i) the distance around the track along its inner edge.
(ii) the area of the track.
Solution: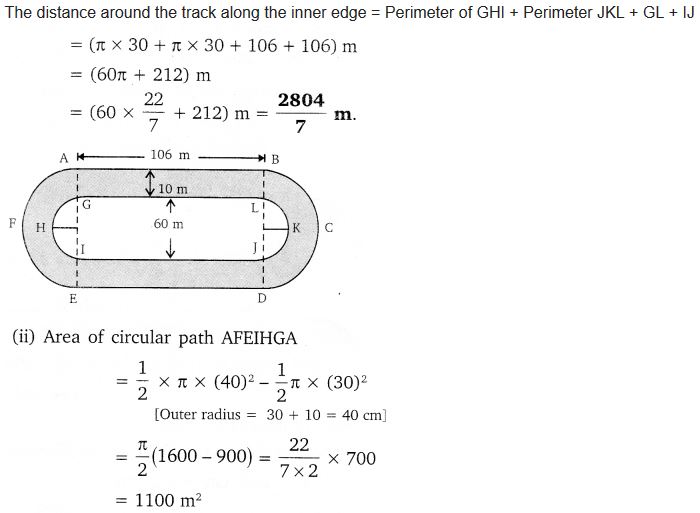

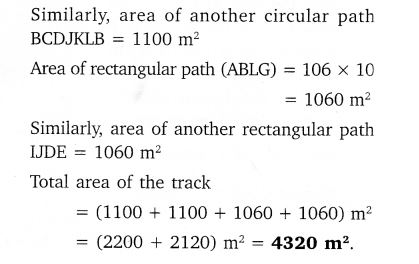
Ex 12.3 Class 10 Maths Question 9.
In the figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region.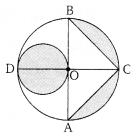

Solution: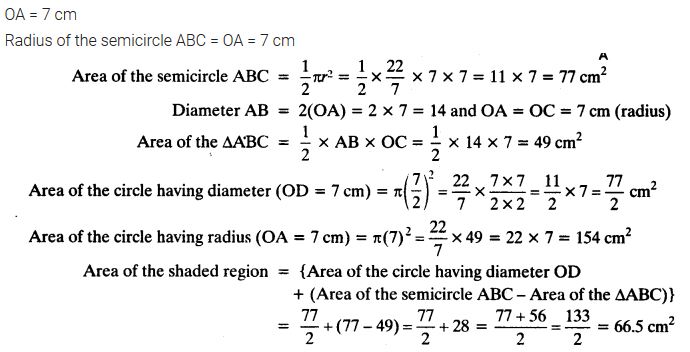

Ex 12.3 Class 10 Maths Question 10.
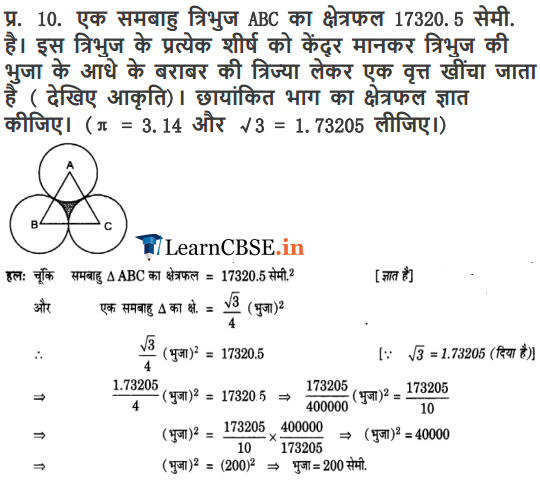
The area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (see figure). Find the area of the shaded region.
(Use π = 3.14 and √ 3 = 1.73205).

Solution: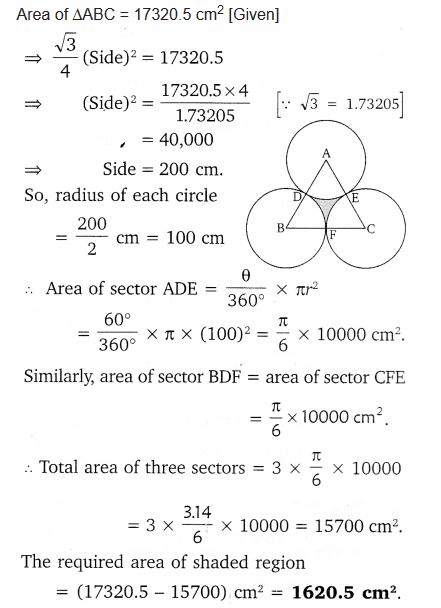

Ex 12.3 Class 10 Maths Question 11.
On a square handkerchief, nine circular designs each of the radius 7 cm are made (see figure). Find the area of the remaining portion of the handkerchief.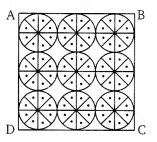

Solution:

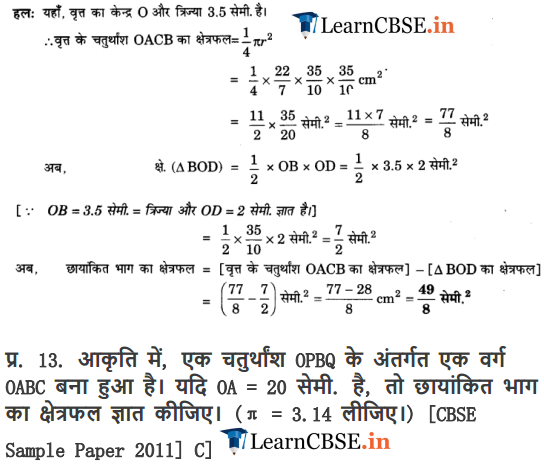
Ex 12.3 Class 10 Maths Question 12.
In the figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) quadrant OACB,
(ii) shaded region.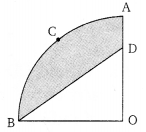

Solution: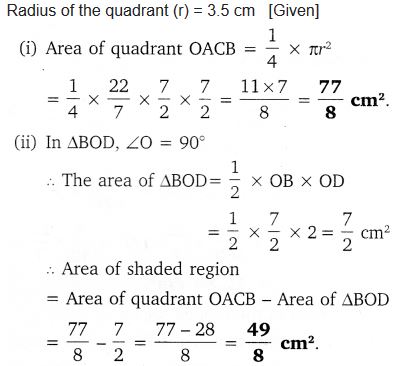

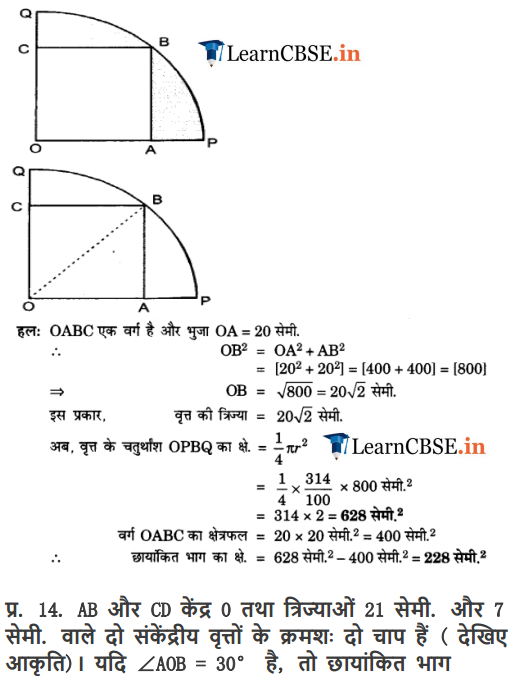
Ex 12.3 Class 10 Maths Question 13.
In the figure, a square OABC is inscribed in a quadrant OPBQ. If OA = 20 cm, find the area of the shaded region. (Use π = 3.14)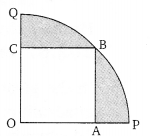

Solution: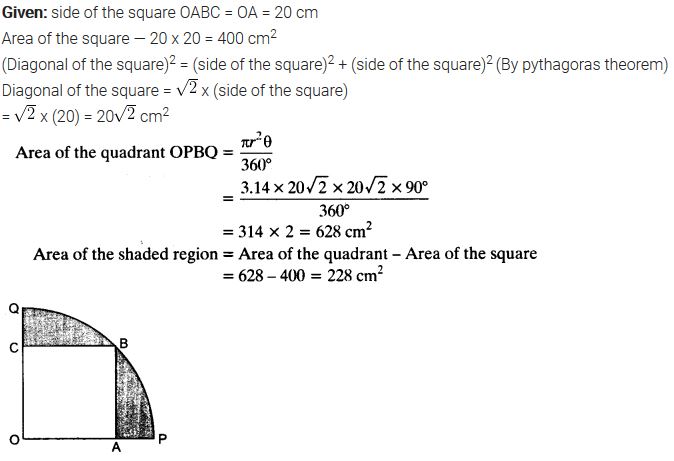

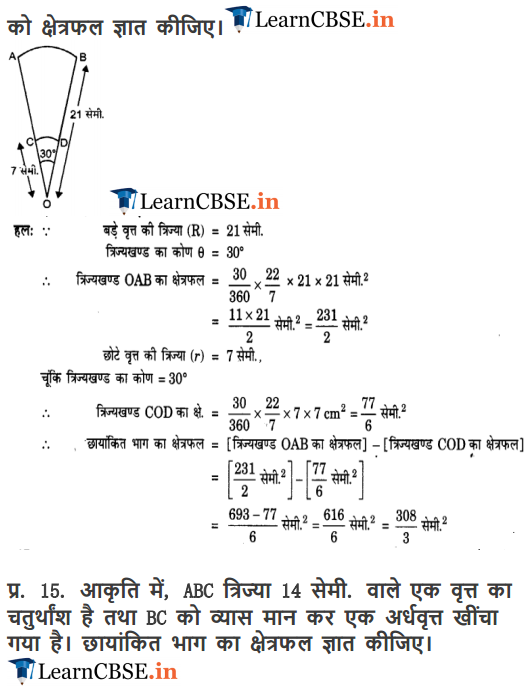
Ex 12.3 Class 10 Maths Question 14.
AB and CD are respectively arcs of two concentric circles of radii 21 cm and 7 cm and centre O (see figure). If ZAOB=30°, find the area of the shaded region.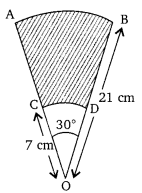

Solution: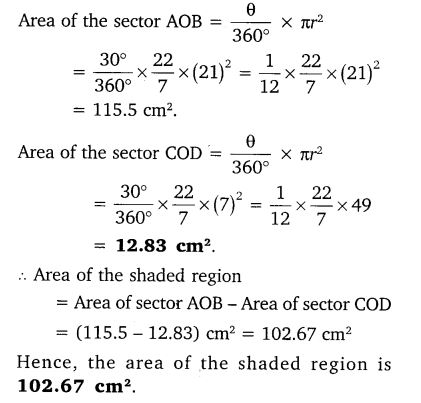

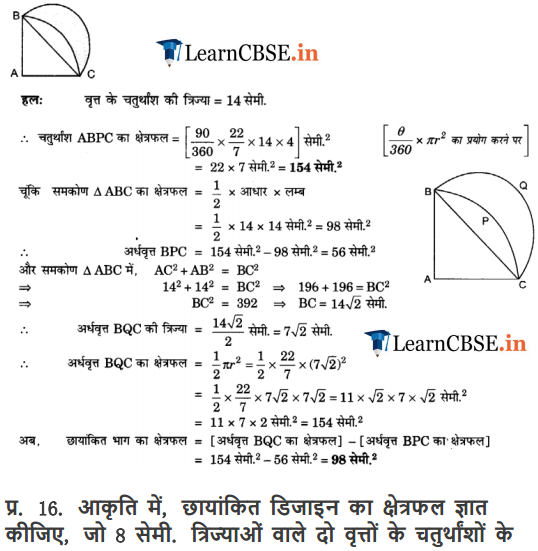
Ex 12.3 Class 10 Maths Question 15.
In the figure, ABC is a quadrant of a circle of radius 14 cm and a semicircle is drawn with BC as diameter. Find the area of the shaded region.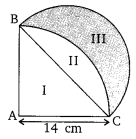

Solution:

Ex 12.3 Class 10 Maths Question 16.
Calculate the area of the designed region in the figure common between the two quadrants of the circles of the radius 8 cm each.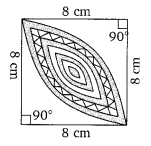

Solution: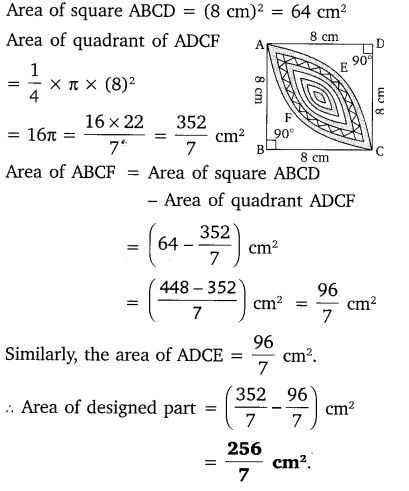

NCERT Solutions for Class 10 Maths Chapter 12 Areas Related to Circles (Hindi Medium) Ex 12.3

Important Questions for Class 10 Maths Chapter 12 Areas Related to Circles

Areas Related to Circles Class 10 Important Questions Very Short Answer (1 Mark)

Question 1.
If the area of a circle is equal to sum of the areas of two circles of diameters 10 cm and 24 cm, calculate the diameter of the larger circle (in cm). (2012D)
Solution:
πR2 = πr21 + πr22
πR2 = π(r21 + πr22) [ r1 = 102 = 5cm, r2 = 242 = 12 cm]
R2 = 52 + 122 = 25 + 144
R2 = 169 = 13 cm
∴ Diameter = 2(13) = 26 cm

Question 2.
The circumference of a circle is 22 cm. Calculate the area of its quadrant (in cm2). (2012OD)
Solution:
Circumference of a circle = 22 cm 2πr = 22 cm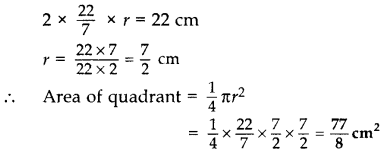

Question 3.
If the difference between the circumference and the radius of a circle is 37 cm, then using π = 227, calculate the circumference (in cm) of the circle. (2013D)
Solution:
2πr – r = 37 ⇒ r(2π – 1) = 37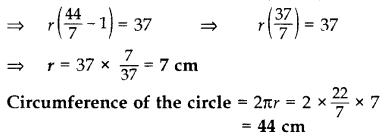

Question 4.
If he is taken as 227, calculate the distance (in metres) covered by a wheel of diameter 35 cm, in one revolution. (2013OD)
Solution:
Radius (r) = 352
Required distance = Perimeter = 2πr
= 2 × 227 × 357 cm = 110 cm or 1.1 m

Question 5.
In Figure , find the area of the shaded region. (2011OD)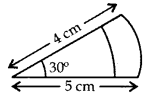

Solution:
Area of shaded region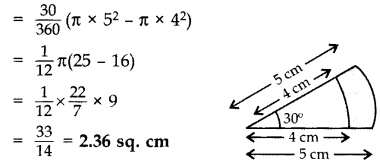

Areas Related to Circles Class 10 Important Questions Short Answer-I (2 Marks)

Question 6.
The length of the minute hand of a clock is 14 cm. Find the area swept by the minute hand in 5 minutes. (2013OD)
Solution:

Question 7.
PQRS is a diameter of a circle of radius 6 cm. The equal lengths PQ, QR and RS are drawn on PQ and QS as PToo RT diameters, as shown in Figure. Find the perimeter of the shaded region. (2011OD)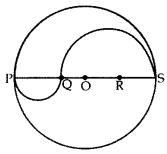

Solution:
Radius OS = 6 cm
∴ Diameter PS = 12 cm
∵ PQ, QR and RS, three parts of the diameter are equal.
∴ PQ = QR = RS = 4 cm
and QS = 2 × 4 = 8 cm
∴ Required perimeter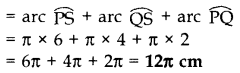

Question 8.
In Figure, a square OABC is inscribed in a quadrant OPBQ of a cirlce. If OA = 20 cm, find the area of the shaded region. (Use π = 3.14) (2014D)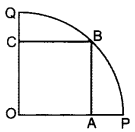

Solution:
Diagonal of the square (OB) = Side 2–√
∴ r = 202–√ cm …[Side of square, OA = 20 cm
∴ θ = 90°
ar(Shaded region) = ar(Quad. Sector) – ar(Square)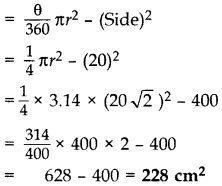

Question 9.
Two circular pieces of equal radii and maximum area, touching each other are cut out from a rectangular card board of dimensions 14 cm × 7 cm. Find the area of the remaining card board. [Use π = 227] (2013D)
Solution: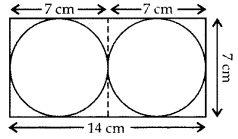

Here r = 72 cm, L = 14 cm, B = 7 cm
Area of the remaining card board
= ar(rectangle) – 2(area of circle)
= L x B – 2πr2)
= 14 × 7 – 2 × 227 × 72 × 72
= 98 – 77 = 21 cm2

Areas Related to Circles Class 10 Important Questions Short Answer-II (3 Marks)

Question 10.
Find the area of a quadrant of a circle, where the circumference of circle is 44 cm. (Use π = 227] (2011OD)
Solution:
Circumference of a circle = 44 cm
⇒ 2πr = 44 cm

Question 11.
Area of a sector of a circle of radius 14 cm is 154 cm2. Find the length of the corresponding arc of the sector. [Use π = 227] (2011OD)
Solution:
Area of sector = 154 cm2

Question 12.
In the Figure, PQ and AB are respectively the arcs of two concentric circles of a radii 7 cm and 3.5 cm and centre O. If ∠POQ = 30°, then find the area of the shaded region. [Use π = 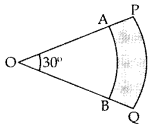227] (2012D)

Solution:
Area of sector with radius 7 cm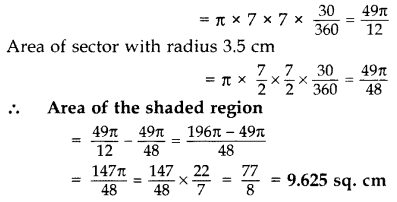

Question 13.
In Figure, a circle is inscribed in an equilateral triangle ABC of side 12 cm. Find the radius of inscribed circle and the area of the shaded region. (Use π = 3.14 and 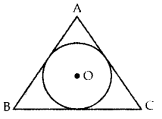3–√ = 1.73) (2014D)

Solution:
Area of an Equilateral ∆ = 3√4 (side)2
Construction: Draw OD ⊥ AB, OE ⊥ BC and OF ⊥ AC. Join OA, OB and OC.
Proof: Area of ∆ABC
= ar(∆AOB) + ar(∆BOC) + ar(∆AOC)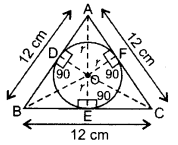

Question 14.
In the figure, PSR, RTQ and PAQ are three semicircles of diameters 10 cm, 3 cm and 7 cm respectively. Find the perimeter of the shaded region. [Use π = 3.14] (2014D)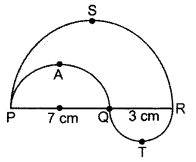

Solution:
Radius of circle QTR = r1 = 32 = 1.5 cm
Radius of circle PAQ = r2 = 72 = 3.5 cm
Radius of circle PSR = r3 = 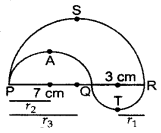7+32 = 5 cm

Perimeter of the shaded region
= πr1 + πr2 + πr3
= π(r1 + r2 + r3)
= 3.14(1.5 + 3.5 + 5)
= 3.14(10) = 31.4 cm

Question 15.
In Figure, ABC is a triangle rightangled at B, with AB = 14 cm and BC = 24 cm. With the vertices A, B and C as centres, arcs are drawn, each of radius 7 cm. Find the area of the shaded region. (Use π = 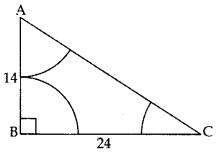227) (2011OD)

Solution:
Let ∠BAC = θ1, ∠ABC = θ2 and ∠ACB = θ3
Area of the shaded region
= ar(∆ABC) – [ar(sector A) + ar(sector B) + ar(sector C)]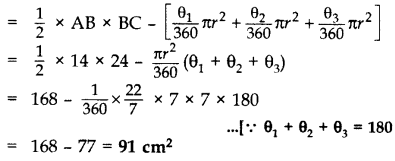

Question 16.
Find the area of the major segment APB, in the figure of a circle of radius 35 cm and ∠AOB = 90°. (Use π = 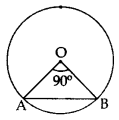227) (2011D)

Solution:
Here θ = 90°, p = OA = OB = 35 cm
Area of minor segment = ar(minor sector) – ar(∆AOB)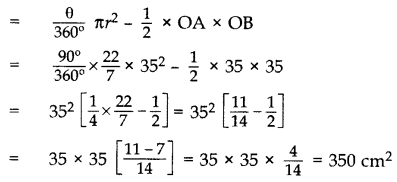

Question 17.
A chord of a circle of radius 14 cm subtends an angle of 120° at the centre. Find the area of the corresponding minor segment of the circle. (Use π = 227 and 3–√ = 1.73] (2011OD)
Solution:
Here θ = 120°, r = 14 cm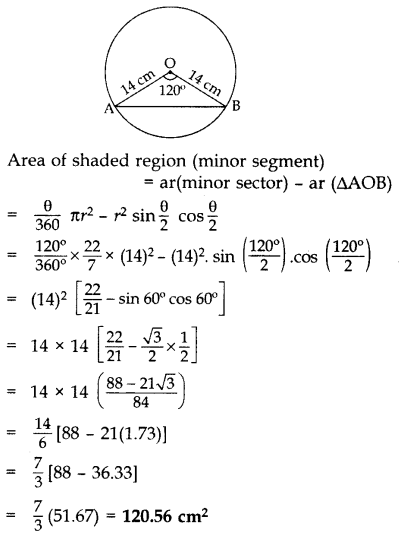

Question 18.
In Figure, a semi-circle is drawn with O as centre and AB as diameter. Semi-circles are drawn with AO and A OB as diameters. If AB = 28 m, find the perimeter of the shaded region. [Use π = 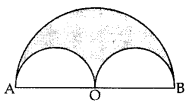227 (2011OD)

Solution: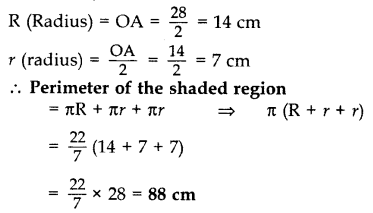

Question 19.
In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find:
(i) the length of the arc
(ii) area of the sector formed by the arc. [Use π = 227] (2013D)
Solution:
(i) Length of the arc: r = 21 cm, θ = 60°
Length of the arc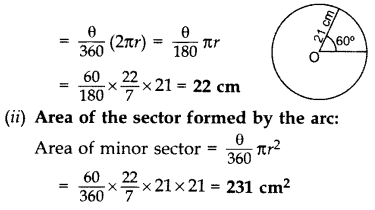

Question 20.
In Figure, two concentric circles with centre O, have radii 21 cm and 42 cm. If ∠AOB = 60°, find the area of the shaded region. (Use π = 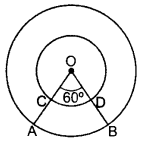227] (2014OD, 2016OD)

Solution:
1st Method: Here θ = 60°
∴ Area of the shaded region = ar (major sector of large circle) – ar (major sector of small circle)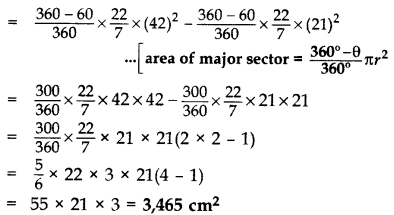

2nd Method: Required area
= ar (large circle) – ar (small circle) – ar (ABCD)
= ar (large circle) – ar (small circle) – ar (minor sector of large circle) – ar (minor sector of small circle)

3rd Method:
Shaded region = ar (large circle)
– ar (minor sector of large circle) – ar (major sector of small circle)

Question 21.
In Figure, APB and AQO are semi-circles, and AO = OB. If the peri-meter of al the figure is 40 cm, find the area of the shaded region. [Use π = 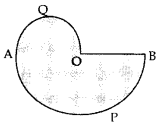227] (2015D)

Solution:
OA = OB …[Given
Let OA = OB = r
AO is the diameter of small semicircle
∴ Radius of small semicircle, R = AO2 = r2
BO is the radius of big semicircle, r
Total perimeter = Perimeter of small semi-circle + Perimeter of big semi-circle + OB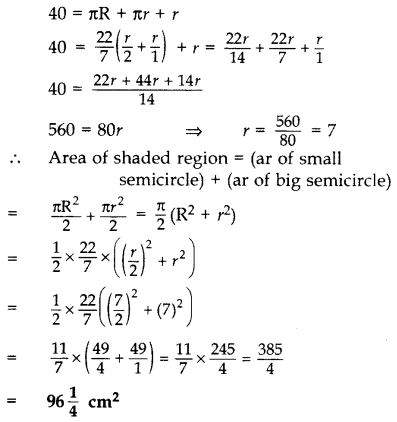

Question 22.
In Figure, O is the centre of a circle such that diameter AB = 13 cm and AC = 12 cm. BC is joined. Find the area of the shaded region. (Take π = 3.14) (2016OD)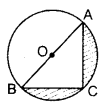

Solution:
∠ACB = 90° …[Angle in a semi-circle
∴ AC2 + BC2 = AB2 …[Pythagoras’ theorem
(12)2 + BC2 = (13)2
144 + BC2 = 169
BC2 = 169 – 144 = 25
BC = + 5 cm

Question 23.
In Figure, are shown two arcs PAQ and 0 PBQuestion Arc PAQ is a part of circle with centre O and radius OP while arc PBQ is a semi-circle drawn on PQ as diameter with centre M. If OP = PQ = 10 cm, show that area of shaded region is 25(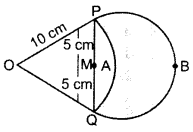3–√=π6)cm2. (2016D)

Solution:
OP = OQ = 10 cm (Tangents drawn from an external point are equal
PQ = 5 + 5 = 10 cm
OP = OQ = PQ = 10 cm … [sides are equal
∴ ∆POQ is an equilatral ∆.
⇒ ∠POQ = 60° …[Angles of an equilateral ∆
Side = 70 cm, r = 5 cm, θ = 60°, R = 10 cm
Area of the shaded region = Area of ∆OPQ + Area of Semi-circle(PBQM) – Area of sector (OPAQ)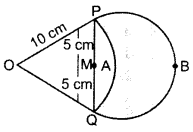

Question 24.
In Figure, O is the centre of the circle with AC = 24 cm, AB = 7 cm and ∠BOD = 90°. Find the area of the shaded region. (Use π = 3.14) (2012OD)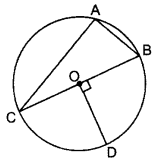

Solution:
∠BAC = 90° …[Angle in a semi-circle
Int rt. ∆BAC,
BC2 = AC2 + AB2 …(Pythagoras’ theorem
= 242 + 72
= 576 + 49 = 625
BC = 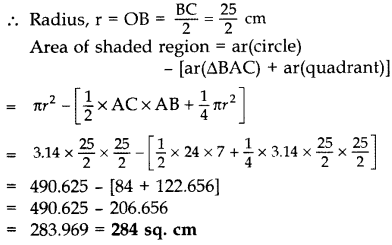625−−−√ = 25 cm

Question 25.
In Fig., AB and CD are two diameters of a circle with centre 0, which are perpendicular to each other. OB is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. (Use π = 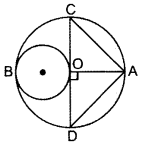227) (2013D)

Solution:
Here, r = 7/2 cm, R = 7 cm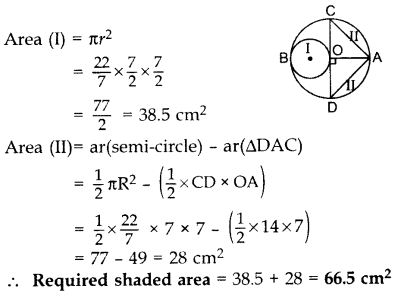

Question 26.
In Figure, APB and CCD are semi-circles of diameter 7 cm each, while ARC and a BSD are semi-circles of diameter 14 cm each. Find the perimeter of the shaded region. [Use π = 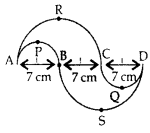227] (2011D)

Solution:
BC = R = 7 cm
AB = r = 72cm
The perimeter of the shaded region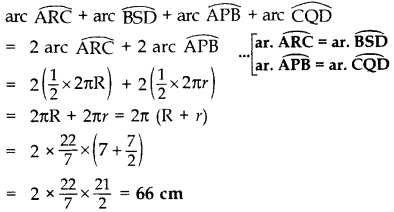

Question 27.
In Figure, ABCD is a trapezium of area 24.5 sq. cm. In it, AD || BC, ∠DAB = 90°, AD = 10 cm and BC = 4 cm. If ABE is a quadrant of a circle, find the area of the shaded region. [Take π = 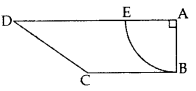227] (2014OD)

Solution:
ar (trapezium) = 24.5 cm2 … [Given
= 12(AD + BC) × AB = 24.5 …(i)
= (10 + 4) × AB = 24.5 × 2
= 14.(AB) = 49
AB = 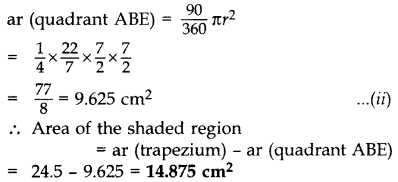4914 = 72 cm = r

Question 28.
Find the area of the minor segment of a circle of radius 14 cm, when its central angle is 60°. Also find the area of the corresponding major segment. [Use π = 227] (2015OD)
Solution:
Here, r = 14 cm, θ = 60°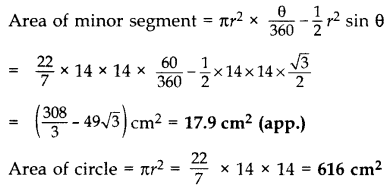

Area of major segment = Area of circle – Area of minor segment
= 616 – 17.9 = 598.10 cm2 (Approx.)

Question 29.
Find the perimeter of the D shaded region in Figure, if ABCD is a square of side 14 cm and APB and CPD are semicircles. [Use π = 227] (2011OD)

Solution: Perimeter of the shaded region
= Circumference of circle + AD + BC
= 2πr + 14 + 14
= 2 × 227 × 7 + 28 ….[. r = 142 = 7 cm
= 44 + 28 = 72 cm

Question 30.
In Figure, OABC is a square of side 7 cm. If OAPC is a quadrant of a circle with centre O, then find the area of the sha- o ded region. [Use π = 227] (2012D)

Solution:
Area of shaded region = Area of Square – Area of Quadrant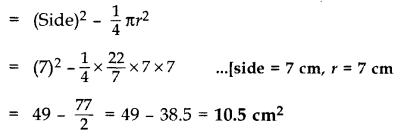

Question 31.
In Figure ABCD is a square of side 4 cm. A quadrant of a circle of radius 1 cm is drawn at each vertex of the square and a circle of diameter 2 cm is also drawn. Find the area of the shaded region. (Use π = 3.14) (2012OD)

Solution:
Area of 4 quadrants (a circle)
= 4 × 14π(1)2
= π sq.cm
Area of the square = (4)2 sq.cm = 16 sq.cm
Area of the circle at the centre = π(r)2 = π(1)2
= π sq.cm
∴ Area of shaded portion
= Area of square – 2 (Area of circle)
= 16 – (11 + r) sq.cm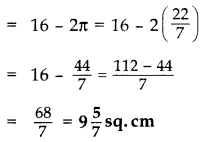

Question 32.
From a rectangular sheet of paper ABCD with AB = 40 cm and AD = 28 cm, a semi-circular portion with BC as diameter is cut off. Find the area of the remaining paper. (Use π = 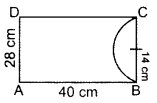227) (2012OD)

Solution:
Length of paper,
AB, l = 40 cm
Width of paper,
AD, b = 28 cm
Area of paper = l × h
= 40 × 28 = 1120 sq. cm
Diameter of semi-circle = 28 cm …(i)
Radius of semi-circle, r = 14 cm
Area of semi-cirice = 12πr2=12×227×14×14
= 308 sq.cm …(ii)
∴ Area of remaining paper = 1120 – 308
= 812 sq.cm

Question 33.
In Figure, find the area of the shaded region, if ABCD is a square of side 14 cm and APD and BPC are semi-circles. (2012OD)

Solution:
Side (diameter) = 14 cm
∴ radius, r = 7 cm
Area of shaded region
= ar(Square) – 2ar(semi-circle)

Question 34.
In Figure, find the area of the shaded region. [Use π = 3.14] (2015D)

Solution:
Let the side of big square = A = 14 cm
Let the radius of circle,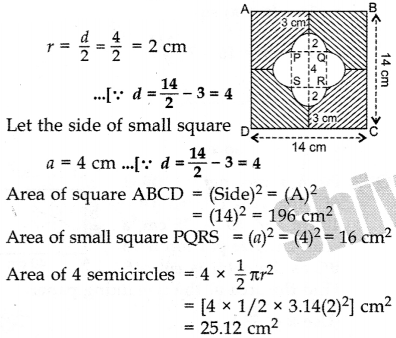

∴ Required area = (Area of big square – Area of small square – Ar. of 4 semicircles)
= (196 – 16 – 25.12) cm2
= 154.88 cm2

Question 35.
All the vertices of a rhombus lie on a circle. Find the area of the rhombus, if the area of the circle is 1256 cm2. [Use π = 3.14] (2015OD)
Solution:
Let r be the radius of circle
In rhombus, AB = BC = CD = AD
⇒ AC = BD = 2r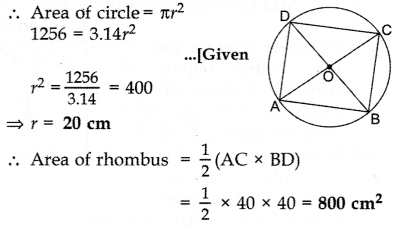

Question 36.
In Figure, ABCD is a square of side 14 cm. Semi-circles are drawn with each side of square as diameter. Find the area of the shaded region. [Use π = 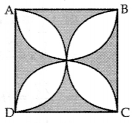227] (2016D)

Solution: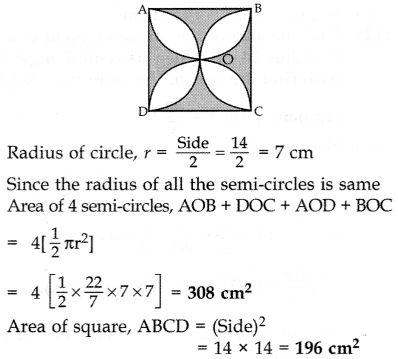

Area of shaded region
= 2(Area of square) – 4(Area of semicircle)
= 2 × 196 – 308 = 392 – 308 = 84 cm2

Areas Related to Circles Class 10 Important Questions Long Answer (4 Marks)

Question 37.
In Figure, arcs are drawn by taking vertices A, B and C of an equilateral triangle ABC of side 14 cm as centres to intersect the sides BC, CA and AB at BZ their respective mid-points D, E and F. Find the area of the shaded region. [Use π = 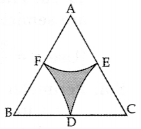227 and 3–√ = 1.73] (2011D)

Solution:
∠ABC = ∠BAC = ∠ACB = 60° … [equilateral ∆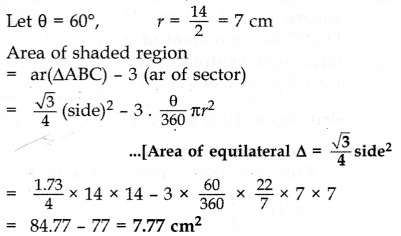

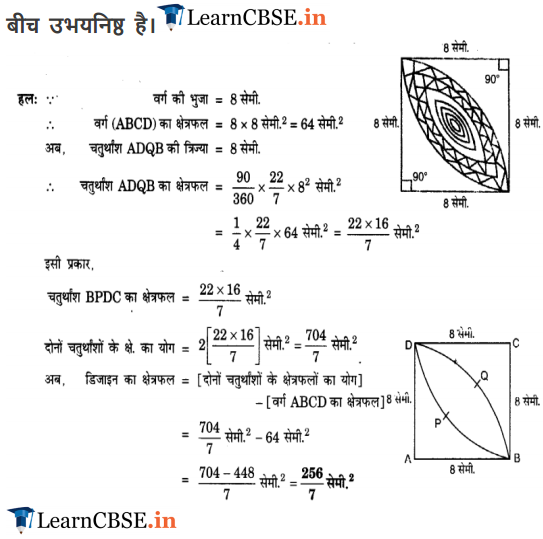
Question 38.
Find the area of the shaded region in Figure, where arcs drawn with centres A, B, C and D intersect in pairs at mid-points P, Q, R and S of the sides AB, BC, CD and DA respectively of a square ABCD, where the length of each side of square is 14 cm. (Use π = 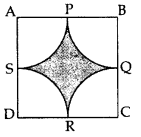227) (2011D)

Solution:
Side = 14 cm, radius, r = 142 = 7 cm
Area of the shaded region
= ar (square) – 4 (ar of quadrant)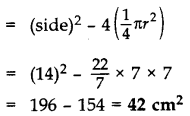

Question 39.
In Figure, three circles each of radius 3.5 cm are drawn in such a way that each of them touches the other two. Find the area enclosed between these three circles (shaded region). [Use π = 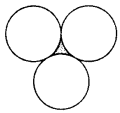227] (2011OD)

Solution:
AB = BC = CA
= 2(3.5) = 7 cm
∴ ∆ABC is an equilateral ∆

Question 40.
Find the area of the shaded region in Figure, where ABCD is a square of side 28 cm. (2011OD)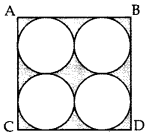

Solution:
Here r = 284 = 7 cm
Area of the shaded region
= ar(square) – 4(circle)
= (side)2 – 4 (πr2)
= (28)2 – 4 × 227 × 7 × 7 = 784 – 616 = 168 cm2

Question 41.
In Figure, an equilateral triangle has been inscribed in a circle of radius 6 cm. Find the area of the shaded region. [Use π = 3.14] (2011OD)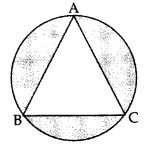

Solution:
Here θ = 3603 = 120°, r = 6 cm
Area of shaded region
= 3(ar of minor segment) = 3[ar(minor sector) – ar(∆ABC)]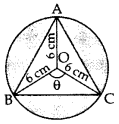

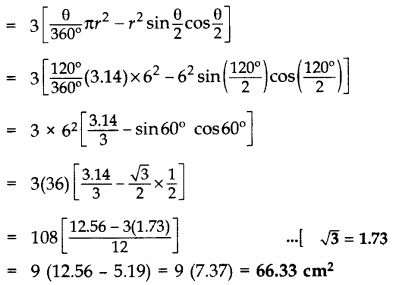
Question 42.
In Figure, ABC is a right-angled triangle right angled at A. Semicircles are drawn on AB, AC and BC as diametres. Find the area of the shaded region. (2013D)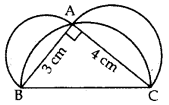

Solution:
In rt. ∆BAC,

∴ BC2 = AB2 + AC2 …[Pythagoras’ theorem
= (3)2 + (4)
= 9 + 16 = 25 cm2
∴ BC = +5 cm … [Side of ∆ can’t be -ve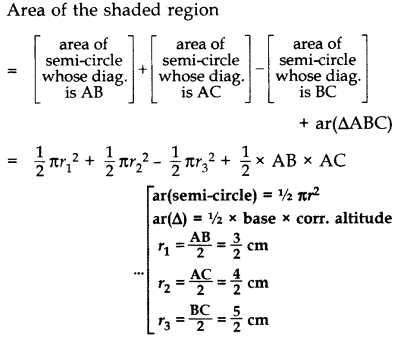

Question 43.
In Figure, PQRS is a square lawn with side PQ = 42 metres. Two circular flower beds are there on the sides PS and QR with centre at O, the inter- section of its diagonals. Find the total area of the two flower beds (shaded parts). (2015OD)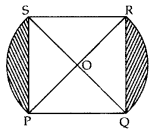

Solution:
PO = 42 m …[Given
PS = QR …[∵ side of square
OR = OP …[Radius of circle
Let OR be the radius of circle = x
So, PR = OR + OP = 2x
Using Pythagoras’ theorem,
⇒(PR)2 = (RQ)2 + (PQ)2
⇒(2x)2 = (42)2 + (42)2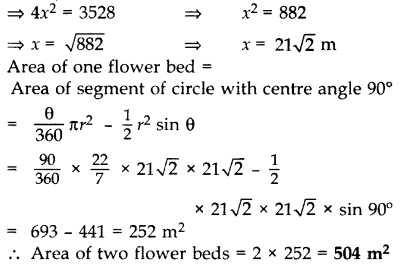

Question 44.
An elastic belt is placed around the rim of a pulley of radius 5 cm. (given figure) From one point C on the belt, the elastic belt is pulled directly away from the centre o of the pulley until it is at P, 10 cm from the point O. Find the length of the belt that is still in contact with the pulley. Also find the shaded area. (Use π = 3.14 and 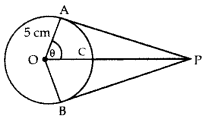3–√ = 1.73) (2016D)

Solution:
∠OAP = 90° ….[Tangent is ⊥ to the center through the point of contact
In rt. ∆OAP,
Cos θ = OAOP=510=12 = cos 60°
∴ θ = 60°
∠AOB = 60° + 60° = 120° …(i)
Reflex ∠AOB = 360° – ∠AOB
= 360° – 120° = 240°
α = 240° …[Let Reflex ∆AOB = α
The length of the belt that is still in contact with the pulley = ADB = lenght of major arc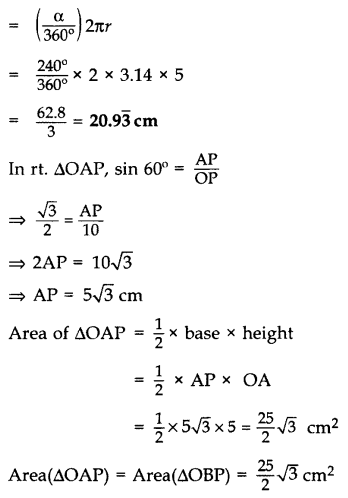

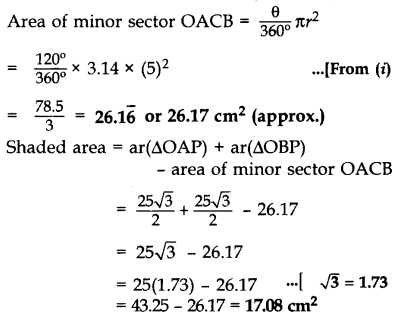
Question 45.
In Figure, is shown a sector OAP of a circle with centre 0, containing ∠θ. AB is a perpendicular to the radius OA and meets OP produced at B. Prove that the perimeter of shaded region is r [tan θ + sec θ + 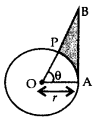πθ180∘ – 1] (2016OD)

Solution:

Question 46.
In the Figure, the side of square is 28 cm and radius of each circle is half of the length of the side of the square where 0 and Oare centres of the circles. Find the area of shaded region. (2017D)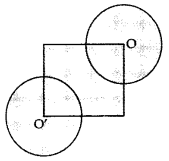

Solution:
Side = 28 cm, Radius = 282 cm = 14 cm
The area of the shade = Area of square + 34 (Area of circle) + 34 (Area of circle)
= Area of square + 32 (Area of circle) …[Area of square = (Side)2; Area of circle = πr2
= (28)2 + 32 × 227 × 14 × 14
= 784 + 924 = 1708 cm2

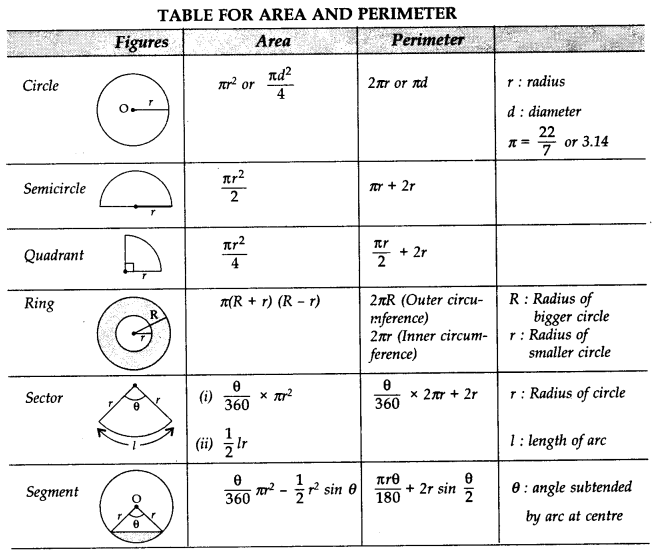
Class 10 Maths Notes Chapter 12 Areas related to Circles

Circumference of a circle = 2πr
Area of a circle = πr2 …[where r is the radius of a circle]
Area of a semi-circle = πr22
Area of a circular path or ring: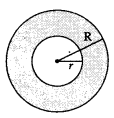

Let ‘R’ and ‘r’ he radii of two circles
Then area of shaded part = πR2 – πr2 = π(R2 – r2) = π(R + r)(R – r)

Minor arc and Major Arc: An arc length is called a major arc if the arc length enclosed by the two radii is greater than a semi-circle.

If the arc subtends angle ‘θ’ at the centre, then the
Length of minor arc = θ360×2πr=θ180×πr
Length of major arc = (360−θ360)×2πr

Sector of a Circle and its Area
A region of a circle is enclosed by any two radii and the arc intercepted between two radii is called the sector of a circle.
(i) A sector is called a minor sector if the minor arc of the circle is part of its boundary.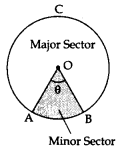
OAB^ is minor sector.

Area of minor sector = θ360(πr2)
Perimeter of minor sector = 2r+θ360(2πr)

(ii) A sector is called a major sector if the major arc of the circle is part of its boundary.
OACB^ is major sector
Area of major sector = (360−θ360)(πr2)
Perimeter of major sector = 2r+(360−θ360)(2πr)

Minor Segment: The region enclosed by an arc and a chord is called a segment of the circle. The region enclosed by the chord PQ & minor arc PRQ is called the minor segment.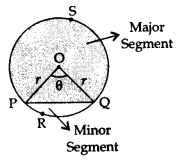

Area of Minor segment = Area of the corresponding sector – Area of the corresponding triangle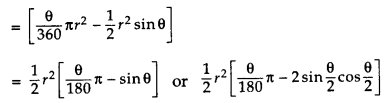

Major Segment: The region enclosed by the chord PQ & major arc PSQ is called the major segment.
Area of major segment = Area of a circle – Area of the minor segment
Area of major sector + Area of triangle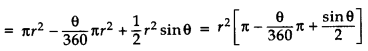

Here Is The Class 10 Maths All NCERT Solutions In Hindi + English With Extra Questions, Notes And Important Questions

• Chapter 1 Real Numbers
• Chapter 2 Polynomials
• Chapter 3 Pair of Linear Equations in Two Variables
• Chapter 4 Quadratic Equations
• Chapter 5 Arithmetic Progressions
• Chapter 6 Triangles
• Chapter 7 Coordinate Geometry
• Chapter 8 Introduction to Trigonometry
• Chapter 9 Applications of Trigonometry
• Chapter 10 Circle
• Chapter 11 Constructions
• Chapter 12 Areas related to Circles
• Chapter 13 Surface Areas and Volumes
• Chapter 14 Statistics
• Chapter 15 Probability